The surface of Venus is around 730K (457°C) – why is it so hot? Although there is still much to learn about Venus the basics have some simple explanations.
Energy Absorbed from the Sun
While earth is 150M km from the sun, Venus is only 108M km away, a ratio of 0.72.
The “solar constant” (as it has been historically called) at the earth is 1367 W/m², and, as energy intensity is proportional to the square of the distance away, or “r²”, the solar constant at Venus is 1367/(0.72²) = 2,636 W/m² – Venus is closer, so it receives more solar energy per m².
The earth has an average albedo of about 0.3, meaning that 30% of the solar radiation is reflected. This is by clouds, by aerosols in the atmosphere, and by the surface of the earth. (Watch out for “The Earth’s Energy Budget – Part Four – Albedo”). If Venus had the same albedo as the earth, the energy absorbed per m² of surface area would be, E = 2,636 * (1-0.3) / 4 = 461 W/m². [corrected – thanks to Bill Stoltzfus for pointing out my mistake]
For an explanation of why the value is divided by 4, see The Earth’s Energy Budget – Part One
This value equates to an “effective radiating temperature” of 300K (27°C). This is nothing like the surface of Venus. [corrected as well – thanks to Bill Stoltzfus for pointing out my mistake]
In any case, it turns out that Venus has a much higher albedo than the earth, with an albedo of 0.76 – meaning that 76% of the solar energy is reflected.
Redoing the calculation, E = 2,636 * (1-0.76) / 4 = 158 W/m² – which equates to an “effective radiating temperature” of 230K (-43°C). The same calculation for the earth gives 255K (-18°C) – see CO2 – An Insignificant Trace Gas? – Part One.
So in terms of a simple energy balance with the sun, Venus should be colder than the earth.
In the case of the earth, as laid out in the CO2 series, the reason the surface of the earth is so much warmer than predicted from simple energy balance is because various trace gases, including water vapor and CO2, absorb the upward radiation from the earth’s surface and reradiate it in all directions. As some of this is downward, the surface of the earth receives more energy than it would without these gases and so it is hotter. See CO2 – An Insignificant Trace Gas? Part Six – Visualization for some more insight into this.
So is Venus so much warmer at its surface because of the inappropriately-named “greenhouse” effect? Or is it for other reasons?
The Venusian Atmosphere
The atmosphere of Venus is quite unwelcoming for us earth-dwellers. The atmosphere is mostly CO2 (97%), with the balance made up mostly of nitrogen (N2), and trace amounts of water vapor and many other gases in minute quantities.
The mass of the Venusian atmosphere is around 100 times that of the earth, and consequently the pressure at the surface of Venus is much higher – at 92bar compared with 1bar for the earth.
Now some people say that the reason for the high temperature at the surface of Venus is because of the high atmospheric pressure and the depth of the atmosphere. For example, Steve Goddard on Wattsupwiththat and echoed by Lubos Motl. This explanation isn’t one that you can find in atmospheric physics text books.
A Quick Review of the Earth’s Surface
This is just to explain a few basics for some perspective.
There’s much of interest in this diagram from Earth’s Annual Global Mean Energy Budget by Trenberth and Kiehl (1997) but we’ll focus on a few key elements for the purposes of this article.
The surface of the earth receives an average of about 170 W/m² from solar energy (with an additional 70W/m² of solar radiation absorbed by the atmosphere). The earth’s surface also receives an average of 324 W/m² of radiation from the atmosphere. So in total the earth’s surface receives about 490 W/m² (annual global average).
Now the average radiation from the surface of the earth is 396 W/m² (or 390 W/m² in the diagram above, which is close enough for our purposes). Convection and conduction remove the balance of around 100W/m². If you take a look at Tropospheric Basics you can see more about the temperature profile in the troposphere (lower atmosphere) and why convection is a more effective re-distributor of heat within the troposphere.
The principal point is that the warming of the air from the surface radiation, conduction and convection causes the air to expand. Air that expands is less dense, and so this air rises, moving heat by convection.
The temperature profile, or lapse rate, from convection can be easily calculated, both for dry air and moist air. Dry air is just under 10°C/km, while moist air depends on the amount of water vapor, but can be as low as 4°C/km. (And the environmental lapse rate, or what we find in practice, is around 6.5°C/km).
So in the case of the earth’s surface, it would be radiating out 490W/m², but for the fact that conduction and convection remove some of this energy from the surface, and then convection redistributes this energy up into the atmosphere.
The Surface of Venus
Energy radiated from a surface is proportional to the 4th power of absolute temperature. This is known as the Stefan-Boltzmann law but visualizing the 4th power of something isn’t that easy. However, calculators are readily available and so if you punch the numbers in you will see that for a surface of T=730K with an emissivity close to a blackbody:
E = 16,100 W/m² – compare this with the surface of earth (288K, 15°C) of around 390 W/m²
This is over 40x the energy radiated from the surface of the earth – for a temperature only 2.5x greater. That’s the real world, very non-linear.. (And note that if the emissivity is not equal to 1, the energy radiated is simply the value above multiplied by the emissivity).
So if we think about the top of atmosphere of Venus, it is radiating round about 158 W/m². This balances the absorbed solar radiation. And yet the surface is radiating 16,100 W/m² – does the high pressure of the Venusian atmosphere explain it?
No
Think about it like this. For the surface of Venus to be radiating at 16,100 W/m² it has to be receiving this energy from somewhere. It receives a maximum of 158 W/m² from the sun (if all of the solar energy absorbed is absorbed in the surface and nothing in the atmosphere).
The explanation from others about a temperature gradient between the surface and the tropopause (top of the tropopause or lower atmosphere) only explains anything when the surface heats the atmosphere from below. In that case the atmosphere heats up, expands and rises – moving energy via bulk movements of air.
Can the atmosphere create heat from pressure and transmit this heat to the surface?
In the case of the earth’s surface, the extra radiation to the earth’s surface (caused by the “greenhouse” effect) heats the atmosphere from beneath and causes convection – with a lapse rate (or temperature profile) of between 4 – 10 °C/km. And convection moves some of this heat from the surface up into the atmosphere. In the case of Venus the argument that relegates the role of the “greenhouse” effect and promotes the role of atmospheric pressure doesn’t have a heat transfer mechanism.
Picture the starting condition where the surface is very cold. What heats it up?
There are three ways of moving heat – radiation, convection and conduction. Conduction in gases is extremely low and anyway the top of the atmosphere is around 230K – if the surface starts off colder what causes heat to flow to the surface to create such a huge emission of radiation?
Convection needs to work by warming a gas from below. Where is this mechanism if the surface is not already heated by the “greenhouse” effect?
And radiation has been ruled out (as the main mechanism) in these arguments from Steve Goddard and others.
How Can the Surface Get so Hot? An Over-Simplified Climate Model
Let’s take a look at the ignored radiation and the super “greenhouse” effect.
How can a surface get so hot from “back-radiation”? Isn’t that just as crazy an idea?
We will take a simple idea – as all models are at their start, just to demonstrate a point. There’s a little bit of maths, unfortunately, but possibly (if you haven’t seen this concept before), the concept might actually seem harder to grasp. Here’s a very simple (and not very realistic) model of a planetary surface and atmosphere (idea from Radiation and Climate from Vardavas and Taylor (2007)):

Simple climate model - atmosphere perfectly transparent to solar radiation, and totally opaque in the infra-red
The surface receives radiation from the sun, S. In the case of Venus this value would be (averaged across the surface), S = 158 W/m².
Now the surface is at Ts and radiates to the atmosphere, which heats it up. The atmosphere is perfectly transparent to solar radiation, but totally opaque in the infra-red and all at one temperature, Ta. Therefore, the atmosphere radiates σTa4 upwards and σTa4 downwards.
Note that “totally opaque” means that no surface radiation makes it through this layer of the atmosphere. In this scenario we can calculate the surface radiation. If you are new to this kind of model, it is easiest to follow the small amount of maths against the graphic. First, using the simple energy balance at top of atmosphere, the outgoing radiation at the top of atmosphere equals the absorbed solar radiation averaged over the surface of the earth:
σTa4 = S [equation 1] – this is the Stefan-Boltzmann law, where σ = 5.67 x 10-8
Second, the surface radiation balances the energy received at the surface – which is from the sun and the atmosphere:
S + σTa4 = σTs4 [equation 2]
Therefore, substituting [1] into [2], we get:
σTa4 + σTa4 = σTs4
2σTa4 = σTs4, or 2S = σTs4
and solving we find, Ts = (2S/σ)1/4
In the case of S = 158 W/m², Ts = 273K
Now effective temperature at the top of atmosphere is 230K, so an opaque atmosphere has increased the surface temperature significantly – but not to 730K. (Barton Paul Levenson has a model like this, commented on in CO2 – An Insignificant Trace Gas? Part Eight – Saturation)
Now with a very optically thick atmosphere, we simply add more and more layers to our model. The equations get slightly harder to solve, but each time we add a new totally opaque layer the temperature rises yet more.
For example, with 3 totally opaque layers the solution to a similar set of equations (with 4 equations and 4 unknowns) is:
Ts = (4S/σ)1/4, or Ts = 328K
It should be easy to see how the surface temperature gets extremely hot from radiation with many layers of opaque atmosphere (yet transparent to solar radiation).
So the Surface Temperature is Infinite, you Dummy!!
Well, if we can keep adding layers, and each one just increases the “back radiation” anyone can see that this can go on forever and the temperature will be infinite!
Obviously the model is wrong..
Not quite (well, if we could keep doing this, the model would be wrong). In the model above we have one totally opaque layer of atmosphere. But once we add multiple layers we are effectively dividing up the real atmosphere and saying that each layer is totally opaque. As we keep sub-dividing the atmosphere into more and more layers eventually they start to get optically thin and the radiation from the layer below will not be completely absorbed.
Radiation from CO2
All the above model does is demonstrate how the presence of significant radiatively absorbing gases can significantly increase the surface temperature. A 97% CO2 atmosphere is different from the model above for two reasons:
- CO2 doesn’t absorb at all terrestrial wavelengths so it isn’t a perfect absorber
- convection will moderate the surface temperature increase – once induced by radiation – as with the earth’s surface
So to calculate the effect of the CO2 atmosphere we have to solve the radiative transfer equations, which you can see in CO2 – An Insignificant Trace Gas? Part Three and Part Five (and the whole series).
These are fundamental equations of absorption and emission, but aren’t really solvable on a pocket calculator – despite so many people appearing to do just that in so many blogs. Note as well that CO2 spectral lines broaden with pressure so that CO2 (and water vapor) become a much more effective absorber in the lower Venusian atmosphere than the earth’s.
And we have to consider that once we have very high temperatures at the surface, convection will begin to move heat more effectively. This essentially moderates the effect of radiation.
But for those who believe that high Venusian atmospheric pressure and the ideal gas laws cause the high 730K surface temperature – they have to explain how the heat is transferred to the surface so that it can radiate at 16,100 W/m².
Real Solutions
One early approach to using real atmospheric physics on this problem was by James Pollack (reference below) in 1969 who showed that that plausible amounts of water vapor and the very high levels of CO2 could explain the high temperatures – using the radiative transfer equations and a convective model.
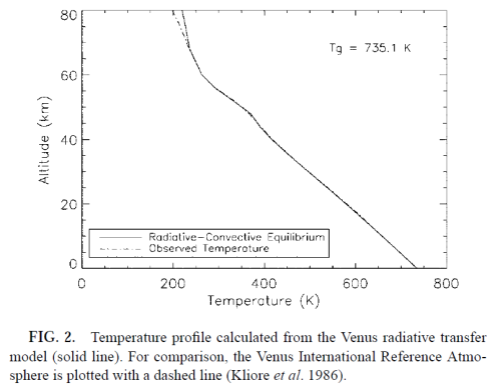
Bullock and Grinspoon (reference below) did this more recent calculation of the temperature profile in the Venusian atmosphere:
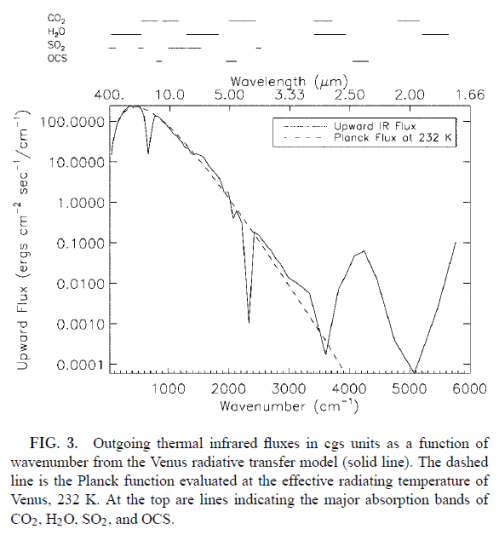
And they note a few possible reasons for the divergence above 70km. The model produces this spectrum of outgoing radiation:
The Planck function for an “effective radiating temperature” of 232K is shown. Note that the much higher levels of flux (in comparison to the 232K curve) demonstrate that at lower wavelengths (higher wavenumbers) the atmosphere is less opaque. This tends to limit further temperature rises, as the presence of any “window” regions allows a higher surface temperature to radiate out efficiently to the atmosphere.
A Mental Model
One mental model for people new to the inappropriately-named “greenhouse” effect is to think about the sun as an internal heat source, and CO2 as some kind of insulator.
Picture an ambient temperature of 20°C and a surface which has a constant internal heat source. As you add more and more insulation around this surface the temperature will keep rising – as heat is less able to flow away from the surface. For a given insulation there will be an equilibrium temperature reached that we can calculate, and it will be a function of the properties of the insulation.
Even though the temperature might reach 100°C or 200°C doesn’t mean that energy is “created” in this model – and this is probably clear to everyone.
Whether or not mental models “work” doesn’t change the realities of physics, but of course everyone wants to understand a subject conceptually.
Conclusion
Venus follows the same physical laws as the earth, so explaining the high surface temperature should be possible, even though many details of the atmosphere of Venus are hidden from us.
Some people who have attempted to explain the high Venusian surface temperature have used the ideas about the relationships between pressure and temperature in ideal gases without the strong “greenhouse” effect of a 97% CO2 atmosphere.
However, these ideas seem to lack a heat transfer mechanism whereby the surface of Venus can radiate at 16,100 W/m². This is the missing element in ideas which eliminate or relegate the role of CO2. In contrast, high surface temperatures in very strongly absorbing atmospheres can be explained using the radiative effects. A simple model can demonstrate very high temperatures, but a thorough calculation does require solution of the radiative transfer equations.
Update – New articles – Convection, Venus, Thought Experiments and Tall Rooms Full of Gas – A Discussion
Reference
A Nongray CO2-H2O Greenhouse Model of Venus, James Pollack, Icarus (1969)
The Recent Evolution of Climate on Venus, Mark Bullock and David Grinspoon, Icarus (2001)



Isn’t convection only important when the actual lapse rate deviates from the adiabatic rate?
As Venus has very little water in its atmosphere then perhaps mechanisms to create deviations in lapse rate are far less than on the Earth?
Assuming there is little convection on Venus, then wouldn’t the lapse rate argument (Temperature is directly proportional to pressure) be a reasonably useful description?
I don’t think this is well put. I’ve set out my own version
The surface air is warmed fairly uniformly. It can’t all rise at once. According to Trenberth et al 2009, “thermals” account for only 17 W/m2. There’s a reason for that. Below the dry adiabatic lapse rate (g/cp = -9.8 C/km) the air is convectively stable. Any air that does become warmer and rise cools at that rate of 9.8 C/km, so if the ambient temperature has a lesser gradient, the heat differential is soon gone. Convection is a minor mode of vertical heat transport (agreeing with Jerry)- latent heat is more significant, but only up to cloud level. IR is the big one.
It’s not really true that the moist lapse rate is easily calculated. It depends on the rate of actual condensation.
I don’t think you really made clear the role of the lapse rate in determining surface temperature. As you say, radiation balance determines temperature at TOA. And then the lapse rate largely determines the gradient from there down. In the case of Venus, it’s a long way down.
So yes, the opacity causes back radiation. But the temperature profile determines how much.
The link to my account of this is here.
Another mistake- sorry. My above comment referred to this quote:
“The principal point is that the warming of the air from the surface radiation, conduction and convection causes the air to expand. Air that expands is less dense, and so this air rises, moving heat by convection.”
Jerry:
No. Convection is important when it moves heat. And if convection occurs then it is moving heat.
I think you are confusing some different processes.
There is convection on Venus and it matches the “dry adiabatic rate” reasonably well.
The “lapse rate argument” is not the same thing as “temperature is directly proportional to pressure”.
Neither of them explain how the surface can be radiating out 16,000 W/m^2.
I don’t understand the argument by Steve Goddard, so I can’t point out exactly where his misunderstanding is. But the ideal gas laws of PV=nRT don’t predict that a high pressure = a high temperature – which is perhaps what you are thinking?
For a fixed amount of gas, if you increase the pressure then if the temperature stays the same the volume must increase, and if the volume stays the same the temperature must increase. That’s all.
Ask yourself – if the surface starts off very cold what increases the temperature? Where does the energy come from?
Science of doom said
Perhaps I mis-stated my point. I think I was trying to say that convection only occurs when the lapse rate is different to the adiabatic lapse rate. Otherwise there would be no energy to drive the process.
A thing I missed in my first post was stating my assumption that – like the proverbial – the sun don’t shine at the surface of Venus. In radiant energy terms the entire sky is a dense, opaque mist that filters energy transport over long time periods so there is little diurnal temperature variation. I could be wrong on this and am happy to be disillusioned.
Science of doom wrote
I rather read that PV=nRT equation as being linear in all variables. That is consistent with one interpretation as you described, but another interpretation is that keeping volume constant means temperature is directly proportional to pressure.
I don’t see how you can say – by implication – that if I get any random parcel of gas of a certain volume that I can’t calculate the temperature from the pressure.
“I think I was trying to say that convection only occurs when the lapse rate is different to the adiabatic lapse rate. Otherwise there would be no energy to drive the process.”
I think that should be “when the lapse rate exceeds the adiabatic lapse rate”. Then the air is convectively unstable – warm air rising cools less fast than the change in ambient temp, and so its bouyancy actually increases as it rises. If the env lapse rate is less (the usual situation), convection is damped, proportionally to the difference.
You can still get natural convection, if there are surface hot spots, for example. But the adiabatic cooling with expansion drains energy from the process.
I think the role of Radiation is often overstated. [I am not qualified to talk about Venus, so I won’t. My remarks are confined to the Earth depicted by the Kiehl & Trenbert diagram.]
The fluxes from the Surface into the atmosphere are:
1. Conduction (labelled as “thermals”) (24W/m^2)
2. Net Radiation (Surface_radiation(S) – Surface_radiation direct_to_space(through the IR window)( R)- Back_Radiation(B), 390-324-40 = 26W/m^2)
3. Evaporated Water(E) (78W/m^2)
So Radiation (from the surface) is responsible for only about one-fifth of the heat flux into the atmosphere from the surface. Conduction is another fifth, and Evaporated Water is by far the most important.
It turns out that if the temperature goes up, the Net Radiation decreases, as the Evaporation increases.
Colin Davidson:
Because longwave radiation to the surface is similar in value to longwave radiation from the surface you think they are less important?
So if we remove the radiation from the atmosphere to the surface we will, therefore, have just a minor effect?
Except now we have lost 324W/m^2 so the surface will end up at.. a very low temperature.
It was a mental sleight of hand.
The upward radiation and the downward radiation are both far larger than convection. The fact that they are similar in value doesn’t negate their importance.
As a less important point, “390-324-40=26 W/m^2” – there is something wrong with this (well, it is mathematically accurate, but that’s about all).
The longwave radiation up from the surface is 390 W/m^2 and the downward longwave radiation at the surface is 324 W/m^2. This is a net of 66 W/m^2.
The fact that 40 W/m^2 of the upward radiation goes through the atmospheric window doesn’t affect the net.
I thank Science_of_doom for his reply.
I intended to make it plain that the flux was absorbed into the atmosphere (I meant into, not through):
“The fluxes from the Surface into the atmosphere are:…
2. Net Radiation (Surface_radiation(S) – Surface_radiation direct_to_space(through the IR window)( R)- Back_Radiation(B), 390-324-40 = 26W/m^2)”
So the calculation is correct.
With Radiation, it is the NET flux that you feel, the NET flux is the only flux doing any work. Whilst the upward and back radiations are very large, it is the difference which affects anything. For example, if we wanted to know how fast a beer from the fridge would warm up, in a vaccuum but in a warm room, we would use the NET flux. [I intend to carry out this experiment, without the vaccuum, shortly…it being Beer O’clock.]
Similarly, if we want to know how quickly the earth loses energy by radiation, we use the NET flux. If we want to know the heating effect of the surface radiation on the atmosphere we must use the NET flux.
When invoking PV=nRT for a planetary atmosphere one must always remember that:
1. P is a constant at the surface determined by the mass of the atmosphere, the surface area of the planet and the value of the acceleration of gravity (fixed by the mass and radius of the planet).
2. T is an independent variable and can potentially have any value.
3. V is a dependent variable and is determined by P and T.
You only get a positive lapse rate (temperature decreasing with altitude) if the atmosphere is more transparent to incoming radiation than outgoing radiation. When the opposite happens, i.e. the stratosphere, the temperature increases with altitude.
DeWitt Payne Said
I must disagree. You can quite easily change volume (V) by changing the enclosure dimensions – such as in a cylinder with a piston. As a result, pressure and temperature will change.
I reiterate my point, that in the equation PV = nRT you can change any variable and the others will follow to maintain the equation.
I also reiterate my point that if you take a volume – say 1 litre – of gas at a lower atmosphere location, it will have higher pressure and temperature than 1 litre higher in the atmosphere.
Planetary atmosphere remember. There are no enclosures.
Sorry – I know this was long ago but the DeWitt reply is something that others seem to confuse. DeWitt states “Planetary atmosphere remember. There are no enclosures” This is both true and false. There is no physical enclosure, but there is gravity acting as a force creating a sort of flexible enclosure. So as far as I understand, you are correct Jerry – when you heat an atmosphere, V and P are variables that will respond to maintain PV=nRT. Proponents of the gas law explaining an atmosphere’s temperature all seem to misunderstand that this formula is about how these variables relate – not how the gas got its energy. But I struggle to articulate this.
Dave,
Gravity is not at all the same as an enclosure with fixed volume. It’s also a constant. When temperature changes, the height of the atmosphere at a given pressure changes, but the pressure at the surface doesn’t change (at least to a first approximation), as it would if the volume were fixed. If anything, the pressure would decrease slightly with increasing temperature because the force of gravity decreases slightly with altitude.
Physicists use the constant volume partial differential equations for thermodynamics because they’re simpler (more elegant). Chemists use the constant pressure equations because those apply to the way chemical reactions are usually run, open to the atmosphere.
Thanks for your post and link to Lubos Motl’s post. I think I’m starting to understand.
Correct me if this is an excessive simplification of your argument, but it seems like you are suggesting that Goddard (and perhaps Motl) are neglecting to take into account the green house effect in providing a mechanism whereby the balance of radiation occurs in the coldest upper atmosphere.
I’m still not clear about how to rectify the multi-layer opaque atmosphere model with the argument about expected lapse rate on Venus (which follows from the argument of atmospheric pressure). If we accept that the balance of radiation is occurring at a certain atmospheric altitude due to sufficient concentrations of greenhouse gases, then couldn’t we argue that the concentration of gas (ie. atmospheric pressure) will tell us what the surface temperature ought to be regardless of concentration of greenhouse gases (so long as there is sufficient greenhouse gases for the atmosphere to be relatively opaque to longwave)?
I will try to articulate this point better after a little more thought.
A second try…
We have initial condition of atmosphere n1 close to surface and is under pressure p1 and has equilibrium temperature T1.
To simulate Venusian conditions we pile a huge amount of atmosphere on top of atmosphere n1 which results in pressure changing to p2.
Without there being any radiation forcing (ie. isentropic process) the temperature of atmosphere above the surface should increase according to T2 = T1(p2/p1) ^[(γ − 1)/γ] where gamma is the heat capacity ratio of the gas.
We didn’t need to increase the input of radiation to atmosphere n1 to create this increase in temperature (in the same way that temperature drop for atmosphere at higher altitude does not necessarily reflect a transfer of thermal energy).
Sorry, beginning of 4th paragraph meant to say “Without there being any change in radiation …”
So what is “scienceofdoom” hiding by his anonymity ?
Anyone who has had even a couple of years of undergraduate math understands the value of orthogonal decompositions . This presentation starts off with the ubiquitous but misleading computation for an unphysical object which absorbs with an “albedo” greater than 0 yet emits with an emissivity of 1 , intrinsically confounding average reflectivity with spectrum . I consider “albedo” an essentially meaningless term except in relation to gray ( flat spectrum ) bodies . For a gray body , it can be unambiguously defined as the reflectivity of the body because it is ORTHOGONAL to spectrum and thus has NO effect on the equilibrium temperature of radiantly heated bodies . This was Kirchhoff great insight 151 years ago . Stefan-Boltzmann does not give just give the temperature of a radiantly heated blackbody , but any similarly heated gray body no matter how dark or light .
It’s not possible to explain a body’s temperature with the standard 1 dimensional “flat earth” image because that is computationally equivalent to a sphere surrounded by a uniform temperature , and by the 0th law of thermodynamics ( and common sense ) the sphere MUST come to the same temperature as that temperature .
Differences from the calculated SB temperature for a gray body can only be explained by differences in the correlations of the object’s spectrum with the spectra of its heat sources and sinks . It’s the ratio of those correlations which determines the ratio of absorptivity to emissivity which may be taken as an operational definition of “the greenhouse effect” . For any set of spectra , this can be exactly computed rather than the non-quantitative word waving about “long” and “short” wavelengths so commonly seen .
If these fundamentals are not understood , there is little point in going further . See my http://CoSy.com for the quantitative implementation of these relationships for gray spheres , and the offer of a cash prize to any student extending them to full spectra .
I don’t presume to understand the vertical temperature structure of atmospheres , nor have I yet seen a coherent quantitative explanation , but for an externally heated sphere , if the energy density on any internal spherical shell is greater than that calculated for the exterior , the explanation is just plain wrong . That is why I continue to find no alternative explanation of Venus’s extreme surface temperature than an internal heat source .
Venus and Hoover are interesting diversions. When do you address the meat of the issue? Positive or negative feedback and the role of non-CO2 factors such as water vapor, clouds, storms, etc.
AGW,
Have you read this post?
This blog started out with very informative and well written presentations of theory and justifications. There was a promise that the meat of the issues affecting earth would be next.
I am not saying these diversions are inappropriate or even uninteresting; I am just asking when the blog will return to the core issues.
Bob Armstrong:
It looks as if others have already answered this question for you, but I can have another go.
The property of absorptivity is a function of wavelength. Solar radiation is at short wavelengths, centered around 0.5um, whereas radiation from a surface like Venus is centered around 4um. 96% of radiation from a 730K surface is above 2.5um while 97% of solar radiation is less than 2.5um.
See The Sun and Max Planck Agree for the sun/earth relationship.
Therefore it is quite likely that the albedo (total proportion of solar radiation reflected) is not related to the emissivity of the Venusian surface.
Here is a handy graph of a few substances – take a look at snow, highly reflective at solar wavelengths, yet highly absorbing at longer wavelengths:
Exactly.
We seem to be talking past each other . Is there any statement I made with which you disagree ?
Blackadder , why do you say a naked Venus would be around 230k ? A gray ball in its orbit will be around 328k .
[moved this from the wrong location]
Mike Blackadder:
For the sake of helping to visualize the processes at work let’s picture Venus with no atmosphere. The surface is quite cold – around 230K.
Now we place the massive Venusian atmosphere above the surface of Venus and let gravity take over. So we “do work” via gravity. And let’s suppose that this increase in pressure creates a higher temperature rather than a smaller volume.
So now we have the lowest part of the atmosphere much hotter. Some heat is conducted into the surface of Venus which was very cold. Let’s suppose that the surface heats up very quickly due to low heat capacity/thermal diffusivity and is quickly in equilibrium with the lower atmosphere at a high temperature.
Some heat is radiated upwards, and with a minimal greenhouse effect this heat will be lost (little or no radiation from the atmosphere back to the surface).
The effect of this cooling is to reduce the kinetic energy of the molecules which therefore, under a constant pressure, reduces the volume.
This is the whole point about the ideal gas laws. They don’t create a continuous source of heat from a constant pressure. If energy is lost from the system the pressure doesn’t regenerate it. If you increase temperature in a fixed volume (like gas in a container) the pressure increases – the thermal energy is kinetic energy which means the molecules “hitting” the walls harder. [Corrected – ] If you increase temperature with a fixed pressure the volume will increase.
Likewise if you reduce temperature the pressure will decrease – OR – the volume will decrease.
Back to our lower atmosphere and the surface radiating 16,000 W/m^2 with little absorption in the atmosphere. The incoming solar energy is 158 W/m^2 and the outgoing radiation is close to 16,000 W/m^2. So the whole climate system cools down. Or does gravity, acting on the gas particles, create an unlimited supply of energy?
Does PV=nRT mean that 158 W/m^2 can come into the system and 16,000 W/m^2 can leave the system indefinitely?
I am commenting with a basic understanding of these concepts so bear with me.
I see your point that an atmosphere can not raise the surface temperature of a planet unless it has greenhouse gases (so long as it is reasonable to assume that the planet will emit radiation like a black body). If surface radiation just passed straight through the atmosphere then perhaps the only effect of the atmosphere on surface temperature would be on the amount of solar that reached the surface. If this was the case on Venus then equilibrium surface temperature would be ~ 330K max.
That seems right to me, and is a useful thought experiment, but I wonder if this is not misleading when we are trying to determine the impact of different factors (ie. greenhouse gas concentration vs. atmospheric pressure). I also wonder if this is so unrealistic of a scenario that people like Motl do not consider such hypothetical atmospheres as a basis for comparison (ie. he would assume that any real atmosphere would absorb long wave radiation from the surface to some extent).
I seems that Lubos Motl’s argument is basically correct that the explanation for high surface temperatures on Venus (relative to Earth) is mostly explained by it having a massive atmosphere (as opposed to a super-greenhouse effect). It is probably correct that we could replace Venus’ atmosphere with a weak greenhouse gas and this would have minimal impact on surface temperature – so long as heat capacity ratio of that gas is similar. Certainly, I think you would agree that if Venus’ atmosphere were somehow comprised of the same atmospheric concentrations as Earth, but was equally massive as now, that Venus’ surface temperature would still be closer to 700K than 300K due to it having such a massive atmosphere.
The argument that high CO2 concentrations on Venus explains its high surface temperature seems like a trick to exaggerate the impact of higher CO2 concentrations on Earth.
Sci ,
Bottom line , I’m saying
Tball : Tgray * ( corr[ SpectrumBall ; SpectrumSun ] % corr[ SpectrumBall ; SpectrumRoSky ] ) * AreaSun % AreaRoSky
I don’t have time to get into all the details extending my algorithm to spectra and , particularly making sure I have appropriate full spectra . That’s why I’m offering a little cash to any student who tackles the task so that we have real , experimentally testable values for any set of spectra of interest .
My question for you is , since you apparently have a good understanding of the relevant spectra , why don’t you calculate precise temperatures from them rather than wave about short and long halves of the spectrum ?
drop that ” * AreaSun % AreaRoSky ” .
It is incorporated in Tgray .
SOD,
I am sorry but you have got it wrong. Steve Goddard on Wattsupwiththat and Lubos Motl had it basically right, although their explanations were not as clear or complete as needed. There is no need for any of the incoming direct (Solar) radiation to reach the ground (and it would not matter if it did), as absorption by clouds would do just as good, and no need for any planet surface based source of energy for the effect of heating on Venus. The incoming radiation that is not reflected is mostly or all absorbed by the atmosphere and clouds. If some reaches the ground, or not, the result is essentially the same. The outgoing long wave thermal radiation has to match the absorbed Solar radiation for the equilibrium case. The atmosphere has to have some greenhouse gases to raise the location where the tropopause occurs to near the top of the atmosphere. i.e., the greenhouse gas sets the temperature for equilibrium to space to near the TOP of the lapse rate location. However, it does not change the location of the tropopause much if you greatly change the amount of greenhouse gas once you have even a modest amount. Note that Venus has 236,000 times as much CO2 as Earth, and Earth has enough greenhouse gas (water vapor and CO2) to have the tropopause above most of the atmosphere. Also note that the upper part of Earth’s atmosphere has already lost most of the Water vapor to clouds or ice crystals, so the fact of water vapor on Earth does not make up for the huge CO2 difference. The radiation both upwards and downwards is nearly balance below the tropopause for an optically thick atmosphere (by definition), and does not matter beyond the final effect at the tropopause. However, due to the variation of incoming Solar intensity at different latitudes, and due to some planetary rotation, there will be convection and currents up and down in the atmosphere. The adiabatic compression and expansion resulting is by far the main source of the large temperature variation from the surface. This lapse rate resulting can vary if there is a phase change, or different composition with altitude, but is basically from g/Cp. The large density of the Venus atmosphere IS the main cause of the high temperature, and if 99.99 percent of the CO2 were replaced by Argon (clearly not a greenhouse gas), and if the albedo even changed a lot, the surface temperature would only change a few degrees. The key point is that the lapse rate is due to the g/Cp to a first order, and the location of the tropopause was moved up from the surface by the fact of a modest amount of some greenhouse gas. The change in location of the tropopause is second order with more greenhouse gas as long as the mass and density of the atmosphere are nearly constant.
SOD,
I see where you got your way of looking at the problem. I worked on a problem using multiple separated layers of reflective foil for a radiation barrier in space or in a vacuum. If the main transport of the energy was radiation, you would be correct. The more the layers, the better the radiation insulation. However, this does not hold up in a thermally conducting and convecting gas. It does not matter that the radiation back and forth between layers is very large, the net radiation transfer between layers is small if the layers are close enough together. The difference between two very large but nearly equal values is still small. The dominant net heat transport through the Venus atmosphere is convective vertical flow.
Leonard Weinstein:
So the surface radiation = 16,000W/m^2.
Absorbed solar radiation = 158W/m^2.
The atmosphere can be non-radiatively absorbing.
And the planet won’t cool down?
How come the surface doesn’t cool?
The GHG view and Goddard’s “Thick Atmosphere” view may be compatible. Anyway, Goddard is by no means the first person to come up with the idea. See:
http://www.countingcats.com/?p=4745
Leonard Weinstein suggests that it would make little difference to surface temperatures on Venus if one replaced the CO2 (a GHG) with Nitrogen at the same pressure. He gets my vote as I came to a similar conclusion using Helium (with its simpler absorption spectrum) instead of Nitrogen:
With dry Helium the lapse rate would be 1.9 degrees Kelvin/km so temperature would fall much more slowly with altitude. If there were no other gasses present most of the long wavelength radiation would pass thorough the atmosphere and into space. The surface temperature would therefore be determined primarily by Stephan’s law. Assuming a surface albedo of 0.3, I accept your estimate of 359 Kelvin for the surface temperature.
Now add sulphuric acid to the atmosphere at concentrations similar to measurements discussed by David Grinspoon (http://www.funkyscience.net/).
After adjusting for the planet’s albedo the mean radiative temperature is 288 Kelvin and this temperature occurs at a height of ~50 km. See “Temperatures within Venus’s atmosphere”, Jenkins 1995:

The sulphuric acid would form clouds and vapour layers which would absorb outgoing long wavelength radiation, thereby allowing the “Greenhouse Effect” to operate. However, the cloud layers would be at ~550 km instead of ~50 km owing to the low density of Helium (4 vs. 44). These clouds would be radiating to outer space at a temperature of ~277 Kelvin (owing to the increased radiative diameter of the planet).
With a Helium/sulphuric acid atmosphere I would therefore expect the surface temperature of Venus to be as high as 1,322 Kelvin (277 + 1.9*550). As measured adiabatic lapse rates can be significantly lower than the “Dry” rate, the temperature could be as low as 900 Kelvin.
You need absorbing gasses AND the adiabatic lapse rate in the troposphere to make the “Greenhouse Effect” work.
Please make allowances for the fact that this is back of the envelope stuff by someone who is more comfortable with high energy gamma sources than IR radiation!
This is a good post. Hopefully we don’t need to hear this “pressure causes super hot temperatures” meme anymore. The explanatory power of the greenhouse effect is well established in the field of astrophysics and climatology and very few interesting climate problems (snowball Earth, faint sun, Venus/Mars evolution, Eocene, glacial/interglacials, etc) can be explained without it. Both Bob Armstrong and Leonard Weinstein have written essentially the same erroneous stuff on my recent post on Venus. Continuing to repeat the same things is not going to make the points any more valid.
One of the things that I have tried to emphasize in my own postings of the greenhouse effect, a concept that cannot be appreciated in the simple “layer model” is that a convective troposphere and the establishment of the adiabat is just as important for understanding the greenhouse effect then is the presence of IR absorbing molecules. Personally, I think the “layer model” is a useless description and cannot come to grips with the spectral dependence and thermal structure of the atmosphere which is crucial for interpreting graphs of outgoing spectra, and in turn, for understanding how the GHE bites off areas under the Planck curve and upsetting radiative equilibrium. Leonard claims that the small amount of solar radiation (~ 160 W/m2) that reaches the Venusian surface does not matter, yet if you replaced this surface absorption with atmospheric absorption then it is possible for a deep layer to become isothermal and substantially cool the planet. Despite my criticism of the layer model, it is actually somewhat instructive to revisit doom’s picture and do calculations for a case where the atmosphere is not transparent to the incoming sunlight. For a geo-engineering detour, you can use a simplified model of this sort to see that even if you keep the surface temperature fixed for a given change in the partitioning of radiative fluxes, you don’t hold the atmospheric temperature fixed, so that has interesting implications for stability and so forth.
OTOH let’s not give the impression the other molecules are irrelevant to everything. Note that it is possible for a pure-diatomic molecule atmosphere to generate a meaningful greenhouse effect if collisions between the molecules become frequent enough, as is the case in very dense atmospheres. It is also possible to substantially heighten the influence of a relatively small greenhouse effect (e.g., 280 ppm CO2) with a heightened background concentration of diatomic molecules. In fact, in Carl Sagan and Muller’s original paper on resolving the faint young sun, the amount of greenhouse gases needed to get their planet just above freezing added to another 1 bar of N2 produced unacceptably hot conditions. On the other hand, Mars which is some 95% CO2 but has virtually no atmosphere only produces a few degrees of greenhouse effect.
There’s lots of other ingredients to the problem. Water vapor for example is a trace molecule on Venus but still important for getting its temperature right. CO2, when thick enough (like Venus) also has a substantial cooling component. Even if you removed all the sulfur clouds from Venus its albedo would still be quite high due to the Rayleigh scattering from CO2. For planets a long way away from the sun which have large enough atmospheres this is very important for establishing the outer edge of the “habitable zone” which astronomers use to determine the range of orbital distances which are conducive to liquid water.
Despite these complexities, you still need CO2 to fundamentally complete the picture of Venus’ hot temperatures since it makes the planet virtually opaque through a wide range of frequencies. The radiation to space can only emanate from a very thin top layer of the atmosphere, with the ratio of the radiating pressure to the surface pressure being rather close to zero, and the difference between the upward surface radiation and the outgoing TOA radiation very large due to the optical thickness. This is not a physically plausible case for a pure Argon atmosphere, and any suggestion that it is comes from ignorance or attempts to confuse.
gallopingcamel,
The emission to space is an integrated quantity which depends on the optical depth from the surface all the way to the top of the atmosphere. Look up the Schwartzchild equation. Putting a good absorber very high up where its cold and then extrapolating down an adiabat to the surface and claiming that now its very hot doesn’t cut it.
“How come the surface doesn’t cool?”
http://www.cosmosmagazine.com/node/1658
cohenite:
From your link you are suggesting that there is an internal, geothermal source of heat.
That would be an equally good way of ensuring that the surface of Venus stayed hot (if it was true).
The essential point of the subject is heat transfer 101.
– An internal heat source into the surface of Venus is one solution to the problem.
– The “super greenhouse” effect from a highly opaque atmosphere of CO2 and a little water vapor is another solution.
Both of the above provide a mechanism whereby the surface can keep radiating at 16,000W/m^2.
The ideal gas laws: high pressure = high temperature from PV=nRT – scores very low on heat transfer basics.
But if it were internal heat source and not GHG, then the IR emitted to space would be 16,000 W/m2 and not 160. That would have been noticed.
Ok Nick I’ll bite, incoming or absorbed solar radiation is 158w/m2 and outgoing is 160w/m2; whether the surface is heated down or from beneath won’t change that, will it?. And what is being overlooked here is how Venus got its atmosphere in the first place; overturning, as suggested by O’Neill, is one explanation; similtaneously providing the atmosphere and the heat.
No, there’s 16000 W/m2 radiated at the surface. If that’s from an internal heat source. that heat has to get out. It must be emitted as IR, else total meltdown.
But if it’s from the 160 W/m2 from the sun, with the temperature enhanced by GHE, outward IR is still 160 W/m2.
If you lie uncovered on a cold night, you emit 100 W to the environment and shiver. With a doona, you are warm, but still emitting 100W. If instead you equip your bed with a 1000W electric blanket, you’re warm too, but emitting 1100 W to the environment.
Hang on, isn’t the 158w/m2 the external radiation from the sun which reaches the surface and the 160w/m2 which leaves from TOA; they’re balanced so the 16000 w/m2 can heat the surface and the atmosphere?
No, if it’s 16000 W/m2 added to the system at the surface, that heat has to leave the planet as IR.
SOD,
You again missed my main point. You do need a long wave absorbing atmosphere to keep from cooling the surface. The point is that 0.0001 times as much CO2 would do the job almost as well (only a few degrees final difference) as the present amount if the mass of the atmosphere were the same. THAT IS STILL 23 TIMES AS MUCH CO2 AS EARTH. The mean absorption distance is a small fraction of the distance to the tropopause, so the direct outgoing radiation is still blocked from the surface out. The multiple absorptions and re-radiations end out eventually radiating to space from the tropopause. I agree some greenhouse gas is needed. However, it is still not the direct cause of most of the temperature rise.
Leonard,
You keep waving around this 0.0001 figure – do you have any quantitative basis for that?
It’s well recognised that extra GHG has a tapering effect – hence the log rule for CO2 on earth. But it can be quantified – you can’t just make up numbers.
Chris,
You also read into my statements what you want to read rather than what is there. I never said that a PURE Argon or Nitrogen atmosphere would still have a hot surface, I said that a relatively small fraction of greenhouse gas is needed to make the atmosphere opaque to the surface outgoing radiation. However, once the level of greenhouse gas is enough to move the location of the part of the atmosphere radiating to space (tropopause) above most of the atmosphere, adding a higher fraction of greenhouse gas only changes the temperature a relatively small additional amount. For example. Earth has 390 ppm CO2, and the calculated DIRECT effect of doubling this level would raise the temperature by 1.2 degrees C. If the atmospheric CO2 increased by a factor of 1000X (but total atmospheric mass remained the same), the DIRECT CO2 caused temperature at the surface would only increase by about 12 degrees C. Once a greenhouse gas is sufficient to raise the location of radiation to space mainly to a high level in the atmosphere, adding a lot more greenhouse gas has a second order effect.
chris colose,
You say:
“The emission to space is an integrated quantity which depends on the optical depth from the surface all the way to the top of the atmosphere.”
I can’t disagree with that but it does not take much in the way of complex molecules to make the atmosphere essentially opaque to outgoing IR from the surface. The small amount of sulphuric acid vapor described by Grinspoon et al. is more than enough to achieve this.
Leonard Weinstein points out that adding a huge excess of GHGs makes very little difference when the atmosphere was already opaque owing to H2SO4 (vapor and clouds) or other gasses.
While I respect your greater expertise, my simple model that assumes that the radiative layer is essentially the top of the Venusian clouds, is a good starting point. I contend that more complex models backed up by super computers will have a relatively minor effect on the calculated surface temperatures.
“The atmosphere is mostly CO2 (97%), with the balance made up mostly of nitrogen (N2), and trace amounts of water vapor and many other gases in minute quantities.”
There is a great debate currently raging on Venus. The Venetians invented the fuel cell about 150 years ago. The fuel cell became the primary source of power that has driven their industrial revolution. Unfortunately, the fuel cells give off water vapor. Many Venetian climate scientists predict global warming forced by increased water vapor. They are pushing for alternative technologies such as the internal combustion engine which emits CO2 instead of water vapor. A small increase in the already dominant CO2 would be insignificant and would not force warming. Skeptics point out that water vapor is an insignificant trace gas that could not possibly cause warming. Others say that even though water vapor can theoretically cause warming in a static atmosphere, the forcing is pretty much cancelled out by negative feedbacks.
Leonard, gallopingcamel;
Be aware that the level which controls the “emission height” of the planet is very much dependent on the wave number. Directly at the 667 1/cm band, even the stratosphere is strongly absorbing. An ideal greenhouse gas would be strongly opaque to IR throughout the whole spectrum, and if CO2 acted as a grey gas it would have a lethal effect upon doubling. On Venus, which radiates primarily at different regions, peaking near the 2450 1/cm region, Venus has a peak absorption area between 2300 and 2400 1/cm and with different absorption characteristics than on Earth. This includes strong continuum effects from pressure and the importance of weak lines which are not relevant to Earth. Further, the “thinning and cooling” argument which raypierre has emphasized at RC is relevant to Venus, which was my original point about only the top thin layer of Venus letting radiation out to space. The logarithmic relationship between forcing and CO2 is only a valid approximation over a relatively narrow range of conditions which are Earth-like. It’s just bad reasoning to assume you can extrapolate a “1.2 K/doubling on Earth” all the way to removing the bulk of CO2 out of the Venusian atmosphere and getting a minor effect.
All of the experts and textbooks remain uncorrected.
cohenite,
Nick’s point is that if there’s an internal source of energy, then the radiation flux out has to be greater than the radiation flux absorbed by the size of the internal energy flux. If your numbers are correct at 158 in and 160 out, then the internal source of energy flux is 2 W/m2. That could contribute to the high surface temperature of Venus, but only if there’s a strong greenhouse effect slowing the loss of heat from the surface. Even then, it could only account for less than 0.5 percent of the temperature difference [ (160/158)^0.25 = 1.0031].
Ah, I replied to Nick before I saw your comment DeWitt; I guess my point is there is obviously a lot of internal heat expressed at the surface of Venus in a way which is fundamentally different and much greater then the volcanic transfer on Earth. Surely that must be contributing to the surface and atmospheric heat in a way which is different from the typical backradiation greenhouse effect?
chriscolose,
Planck and Shreodinger had a “failure to agree” over the nature of light. Particles or Waves? It turned out that both were right. Professor Gilbert Stead summed it up in doggerel:
QUOTE
There would be a mighty clearance,
We should all be Planck’s adherents
Were it not that interference
Still defies h “nu”
UNQUOTE
Your “Greenhouse Effect” theory does not work without the adiabatic lapse rate which allows the radiative layers to be cooler than the surface of a planet. Make peace with Goddard and Lubos Motl.
Everyone relying on the lapse rate and PV=nRT to explain the surface temperature of Venus or the Earth don’t appear to understand why the lapse rate is driven to the adiabatic rate in the first place. In the absence of radiation absorption/emission by the atmosphere, the lapse rate would be very small. If one could make the atmosphere infinitely viscous so no convection could occur, the lapse rate would increase to greater than the adiabatic rate. More heat is lost by radiation to space at any given altitude in the troposphere than is absorbed from radiation from below. So for radiative transfer only, the temperature at any altitude would have to decrease until the flow balanced again. But in the real atmosphere, any increase in the lapse rate above the adiabatic rate causes convection to make up the difference.
DeWitt,
The lapse rate does NOT mainly depend on radiation and/or absorption of the atmosphere. Due to variation in absorbed radiation at different latitudes and due to planet rotation, the surface and or atmosphere is heated at different rates. This results in circulation and vertical mixing. The lapse rate then comes direct from g/Cp with possible variations added if clouds and condensible gases are present (dry vs wet lapse rate). If there were no greenhouse gases or condensible vapors (say 100 percent argon), the heating would all occur at the surface, and all radiation to space would occur at the surface also. There would still be a lapse rate with a colder upper atmosphere due to the adiabatic cooling with increase in altitude. However, the temperature would go down from the surface temperature and there would not be a tropopause, just continual dropping temperature at the lapse rate until the region affected by Solar ions became dominate. The heat would be transported mainly by convection (with a much lower level of conduction) once the conduction from surface to gas occurred. The net transport of heat would be from low latitudes to high latitudes at all altitudes. The temperature at all altitudes would vary with latitude due to the varying surface temperatures.
Look guys, the general region where almost all of Venus’s outgoing radiation (matching the absorbed Sunlight) occurs is in the upper atmosphere at about the tropopause level. This level is about 95 km. the KNOWN lapse rate for Venus is very constant to almost that altitude at about 7.8 degrees C per km. This is almost exactly the value of g/Cp for Venus and CO2 (slight difference occur due to slight condensible SO2 and small trace other gases). There is no room for any other radiation effects (to 1st order) other than the effect of moving the location of the outgoing radiation at a high altitude. The surface temperature is accurately given by the rise calculated from lapse rate plus the value at the tropopause! The effective location of this radiation LOCKS the temperature at the upper end of the lapse rate driven curve. If there were no greenhouse gas, the surface driven radiation would LOCK the temperature of the lapse rate driven curve to the ground, but the temperature drop rate would be the same until the value of Cp changed with temperature, or until the gases started condensing. If the increase in greenhouse gases moved the height of the radiation going to space, this would change the surface temperature, but in the case of Venus, the change would be relatively small for a huge change in greenhouse gases. The radiation, conduction, and convection do not matter beyond being necessary to drive the process of mixing so that the lapse rate occurs. The only place the radiation details matter other that the fact that they are significant to drive the location of final radiation is THE UPPER ATMOSPHERE LOCATION OF THE OUTGOING RADIATION, which would only vary a small amount for huge differences in quantity greenhouse gases.
But Leonard, please quantify. And explain – so what?
DeWitt Payne,
Most of the time I tend to agree with you but you are quite wrong about the adiabatic lapse rate. It can be explained quite precisely using thermodynamics.
In the troposphere, convection and conduction are more than sufficient to move the heat around. Radiative transfer is overwhelmed by the two “C”s.
And Earth’s surface temperature if we removed all non-greenhouse gases from the atmosphere (leaving only the ~ 0.5% trace gas concentrations – ie. Earth, but with a tiny 100% greenhouse gas atmosphere)? Should still be 288K if the argument is that only the greenhouse gas concentrations can impact surface temperature.
Another good post, thanks for these!
One very minor comment – the limit of the greenhouse effect is not infinite temperature, but the surface temperature of the source star (5500 K or so for the sun).
The explanatory reason for this is that as the “surface” temperature increases, the thermal spectrum moves to shorter and shorter wavelengths. Once surface temperature starts getting close to the stellar temperature you need your atmospheric layers to start absorbing the same spectral region that is your incoming radiation – but that means the incoming radiation never actually reaches the surface.
And of course the thermodynamic reason is simple: heat only travels from hotter to colder systems, so as the primary energy source the sun must remain hotter than the planetary surface, no matter what the physical arrangement of component parts.
gallopingcamel,
Indeed, the adiabatic lapse rate is equal to the acceleration of gravity divided by the mass heat capacity at constant pressure. That’s the neutral buoyancy point where a kilogram of air can, in principle, be raised or lowered to any altitude and be at the same temperature as the surrounding air. But that’s a limiting value, not a requirement. A lapse rate greater than adiabatic means that the air below is more buoyant than the air above, an unstable situation, and convection will eventually start and continue until stability is restored. But any lapse rate less than the adiabatic rate is stable to convection because the air above is more buoyant than the air below. We see this in the stratosphere where the lapse rate is negative and temperature increases with altitude. In the stratosphere, essentially all heat is transferred by radiation and there is little or no convection. That’s also driven by radiation. The atmosphere in the stratosphere is less transparent to incoming radiation (absorption of UV by oxygen and ozone) than outgoing radiation, the opposite of the case in the troposphere.
A 1D radiative-convective model with a completely transparent atmosphere will be isothermal because, conduction, as slow as it is, will transfer heat until there is no temperature gradient. A 1D model with an atmosphere similar to our own will revert to a lapse rate similar to our own whether it starts out at a temperature above or below the surface temperature. If it’s colder, convection and radiation from the surface will warm it. If it’s warmer, radiation to space, but not convection, will cool it.
There’s a good online resource for Physical Meteorology here:
Click to access PhysMetLectNotes.pdf
(4.2 MB pdf)
I continue to be profoundly unimpressed by the level of understanding of basic physics displayed on both sides of these blog wars .
I’ve really only made one assertion , the proposal that the “greenhouse effect” be defined as the ratio of the correlations of an object’s spectrum with the spectra of its heat sources and sinks . I do hope people understand the mathematical definition of correlation .
No one has either agreed that that is an appropriate definition , or asserted some other equally unambiguous and quantitative definition .
I can only conclude that no-one in this discussion has any quantitative definition of the “green-house” effect , and thus is only playing at physics , not actually seeking experimentally verifiable quantitative understanding .
If you don’t know how to calculate the temperature of a radiantly heated either gray or colored ball , how do you expect to reduce to computation any more complex problems ?
Btw , I see a lot of confusion between energy density , eg , W/m2 , and power , W/m2/second which explains how a well insulated internally heated body may have a high core temperature but radiate at a much lower rate than a black body .
DeWitt ,
That reference looks like it probably has a lot of useful information , but , page 132 , it has that stupid non-physical assumption of a naked planet having an absorptivity less than 1 yet an emissivity of 1 . I see nothing but inevitable confusion building on that ill-defined foundation .
Bob Armstrong:
I do have a policy on this blog which is rarely, but arbitrarily, applied.
That repetition without attempts to engage will start to get deleted.
However, people who are trying to understand get a lot more latitude than people pronouncing.
Earlier you made a similar assertion to this recent one – “stupid non-physical assumption of a naked planet having an absorptivity less than 1 yet an emissivity of 1” and I responded. Others on another blog have also explained the same points to you – which is why I am explaining this arbitrary aspect of blog policy early on.
So instead of re-pronouncing your point of view, you first need to engage with the comment that emissivity and absorptivity are functions of wavelength – and solar radiation and terrestrial radiation are at different wavelengths.
If you don’t understand it or don’t want to engage with it, that’s fine. But your next confident pronouncement of the same flawed statement might be deleted – after all everyone can read what you have already written on this subject.
Arthur Smith ,
Your point about the “greenhouse effect” being limited is important , particularly in discussions like this where no limit appears to be claimed for the possibility of “runaways” .
But the answer is not that an object can reach the surface temperature of its star , which , in the case of the earth is only about one half millionth of the celestial sphere . Rather , it is ( essentially ) when the spectrum of the object matches that of the star , ie , when the correlation of its spectrum with that of its star approaches 1 .
I am really struggling conceptually with downward radiation from greenhouse gases. Pardon me if my figures aren’t accurate, but my understanding is that without a greenhouse effect, the average temperature of the Earth would be 33C colder. Please feel free to correct me here. Regardless of the exact numbers, the Earth would be uninhabitable without the greenhouse effect.
The Earth radiative budget diagram shown above, I understand, is based on sunlight reaching the surface of the earth averaged over the entire surface of globe, not just the half facing the sun, plus LWR projected downwards by the greenhouse effect, once again averaged over the entire surface of the earth.
Could we please discuss how one might experience this downward radiation. The model described above is completely non-intuitive because sunlight does not shine on the entire Earth evenly all over at the same time.
On a bright equinox day, where the ambient maximum temperature is 33C and the minimum average temperature is 20C for 15 days each side on our theoretical test day (The 30 day clause is there in case long-term stability helps simplify the problem).
How would I experience the greenhouse effect over the course of a this day, say at Midnight, 6AM (sunrise) , noon (solar zenith), and 6PM (sunset)? What would I feel? It seems that direct heat from the sun feels significantly warmer on my skin than the 33C ambient temperature of our test day.
I have also noticed that when skiing on, say a -10C degree day, periods of bright sunlight still feel very warm, much warmer than the ambient temperature.
I have read that the Earth, although not an ideal black body, re-radiates energy almost as well. Does that mean that most of the sunlight reaching the Earth is immediately converted to heat and re-radiated almost immediately, so that very little is re-radiated from the surface after sunset? If so, does that mean most of the greenhouse effect occur during the day, and virtually no effect at night?
Are the radiative forcing equations informative in this instance, or only suitable to a theoretical averaged world.
The “runaway greenhouse” is not defined as a point of “infinite temperature” or even when the planetary temperature approaches the solar temperature. This is a straw man. It is a point at which the emission is continually out-gained by the solar absorption until the oceans are completely depleted, at which point radiative equilibrium can once again become established at a hot temperature.
cherry picked:
The model described is the global annual average so is, of course, not intuitive, because no one experiences the average.
Even better than “how do we experience it?” – is “how do we measure it?”
We measure it quite easily and you can see some measurements at an actual location over the course of a day in Sensible Heat, Latent Heat and Radiation (although I think in another post I have already pointed you there).
Of course. On a -10’C degree day (probably at altitude) with the sun nearly overhead you will “experience” perhaps over 1000 W/m^2 from the sun. Whereas you might “experience” perhaps 200 W/m^2 from atmospheric downward radiation.
No, it doesn’t mean that at all. The surface radiates according to its temperature (proportional to the 4th power of absolute temperature).
And the “greenhouse” effect is the atmosphere radiation, which usually cools down slower than the land, but faster than the ocean.
If you know the temperature profile and the concentrations of various gases (primarily water vapor, as it varies the most) then you can calculate accurate values of atmospheric radiation. You can see one example (non-averaged) of this in CO2 – An Insignificant Trace Gas? Part Six – Visualization
A couple of points from firstly Arthur who says:
“heat only travels from hotter to colder systems,”;
with backradiation in mind does that mean that 2 bodies with temperatures Ta and Tb with both above zero temperature but with Ta hotter than Tb will exchange radiation according to SB but heat will only flow from Ta to Tb?
Secondly Chris says about runaway greenhouse:
“It is a point at which the emission is continually out-gained by the solar absorption until the oceans are completely depleted,”
On Venus with surface absorption at ~ 160w/m2 and TOA outgoing ~ the same then apparently Venus is no longer in a runaway greenhouse situation?
cohenite,
Generally the runaway greenhouse effect is a transient state in which the planet is undergoing a transition from an ocean to no ocean. So no, I would not say that Venus is currently in a runaway greenhouse effect state but was in the past. It is however not possible to regulate its CO2 concentration in the manner that Earth can via silicate weathering and it’s at a point in a phase diagram where virtually the whole CO2 inventory is locked in the atmosphere. You can debate whether you’d like to call this “runaway” or “hell” or “bananas” but whatever terminology you’d prefer, it’s very hot, and CO2 is a big part of that.
Leonard Weinstein:
So I think you are right, I did misunderstand your point. However, now I’ve re-read it and your further comments a few times I’m still not sure I have understood you.
Also you appear to have a totally different explanation from Steve Goddard so the fact that you say he has got it “basically right” confuses me further..
Well, using the radiative-convective model with current knowledge of the Venusian atmosphere, Bullock and Grinspoon calculated the temperature profile quite accurately.
Do you think that if the amount of CO2 was only 0.01% of its current value this would still come out the same?
If the calculations run with 0.01% CO2 / 99.99% Argon comes out with a much lower surface temperature would that change your opinion about your current hypothesis?
Or do you believe that the radiative-convective model supports your hypothesis?
Or it is a flawed model?
Even if there was no convection there would still be a temperature profile in the atmosphere of Venus – due to a radiative equilibrium. The lapse rate would just be higher:
Perhaps again I am misunderstanding your point.
I don’t think I can explain the basics on this as well as DeWitt Payne as he has done an excellent job of explaining what creates convection (and it’s not the lapse rate formula, correct though it is).
A high enough “greenhouse” effect needs to heat the surface so that convective over-turning takes place. Do you think this is right? wrong? missing a point?
Mike Blackadder:
Interesting idea.
I don’t think you’ve quite understood the argument though. You need the presence of radiatively absorbing gases to get a high enough temperature. But the atmosphere can moderate this effect via convection.
With little convection due to a tiny atmosphere the temperature may be higher than 288K. I say “may” because this solution “may” be the obvious one, but I would want to see the full RTE solution to be sure.
I’m sure that I don’t understand the argument about how the temperature would be higher. The greenhouse effect only works in heating the planet because it emits radiation to space from upper atmosphere which is cold relative to the surface. ie. In your greenhouse effect illustration we see 390 longwave from atmosphere back to surface and 165 longwave from atmosphere to space.
My hypothesis would be that Earth with tiny atmosphere would have less differential between surface temperature and the altitude where radiation is balanced with incoming solar, and so surface temperature would be lower. What is the alternate explanation?
I think that’s correct. A 288K surface in those circumstances would imply a very great temperature gradient, which would be hard to sustain.
SOD,
The point that was correct by Steve, and the point I support, was that most (not all) of the high surface temperature of Venus is due to lapse rate combined with enough greenhouse effect to move the source of radiation to the upper edge of the atmosphere (which locks the temperature at that altitude). I know the radiation to space is spread over a volume, but I use a nominal height. I have no other comments on the structure of his writeup. I totally agree that different amounts of greenhouse gases and radiation vs convection domination make a difference in the final lapse rate and curves. However, the difference in result is relatively SMALL compared to the very high overall temperature level. A 99.99 percent Argon and 0.01 percent CO2 (plus a trace of water vapor and SO2) atmosphere at the same total mass would only be a few degrees C lower at the surface (probably <30 degrees C lower). Keep in mind this level of CO2 is still 23 times as much as Earth.
If calculation using 99.99 percent Argon and 0.01 percent CO2 give a MUCH lower surface temperature, I would be convinced that I may be wrong. I would like to see the result in either case. Much is a relative thing, but the implication of this site seems to be that it would be several HUNDRED degrees C lower. If it is fact only several 10’s of degrees C lower, then I consider that I am correct. I am not skeptical about everything, so I would give a calculation from the model more than a fair shake. I don’t automatically disbelieve something is valid just because I don’t agree with it. However, keep in mind that even the model may be incomplete due to some incorrect assumption or something left out.
SOD,
Consider that on Earth, the absorption depth of CO2 is 10’s of meters. Venus, with 230,000 times the surface quantity of CO2 has an absorption depth of MICRONS. The temperature gradient is order of 8 degrees C per km. Thus the temperature difference between layers where the radiation is transmitted up, and the upper layer transmits back are only a very small density and temperature difference. The net transmitted radiation is only a fraction of a watt, at most, near the ground, and only becomes significant at higher altitudes where the CO2 density is much lower. Almost all of the energy is transported by convection. The dry convection lapse rate will totally dominate.
DeWitt Payne,
Many thanks for that Rodrigo Caballero treatise you linked. On page 133 he makes my point much better than I could hope to:
QUOTE
The warming effect of the atmosphere, known as the greenhouse effect, is best understood as follows. The atmosphere is opaque in the infrared, which means that the mean emission level is lifted off the ground. The mean temperature at the emission level (i.e. the mean
brightness temperature) must be Te in order for emission to match absorbed insolation. But the atmosphere has a positive lapse rate, and so the temperature at the ground must be
greater than Te .
UNQUOTE
That just moves the question back one level to why does the atmosphere have a positive lapse rate in the troposphere. Take a close look at the graph that scienceofdoom posted above in his reply to Leonard Weinstein. Note the slopes of the radiative only, dry and moist profiles. The deviation of the radiative only profile from isothermal is greater than the dry adiabatic rate. As I said above, the atmosphere has a near adiabatic temperature profile because radiative heat loss drives it to that profile. In the absence of absorption/radiation by the atmosphere, conduction would dominate and the atmosphere would be nearly isothermal.
It is not realistic to talk about conduction being dominant in the troposphere.
If the atmosphere were incompressible the lapse rate would have the opposite sign (cf. water). The Rodrigo Caballero treatise explains the calculations for a compressible gas quite well. See page 40 onwards.
SoD ,
Yea , this has become a pointless discussion . You appear not to be able to understand or be interested in even nailing down the basic equation for radiantly heated no-gray balls altho you clearly have the spectra available to do the calculations if so inclined . You appear not to even be able to understand the rather simple equation I present .
Instead , in the case of the earth , you pull “out of the air” , a ratio of about 0.7 % 1.0 for the ratio of absorptivity to emissivity ( without providing an example of what spectra would generate such a ratio ) , thus producing the meaningless claim of 33k deficit . Marty Hertzberg comes to the same approximately 279k value for a flat spectrum earth , leaving about a 9k deficit , that I do . ( And in conversation he’s seen the problems with his notion that compression answers the Venus issue , and that internal heating is a simpler answer . )
Then , a bunch of you do a bunch of word waving , no experimentally testable computations , claiming that indeed heat may be made to flow from the exterior of a radiantly heated sphere to generate a temperature on its interior greater than that of its exterior . If you can reduce that to quantitative equations , it’s too bad you won’t be able to patent it because surely we can construct analogous spheres here on earth and suck all the energy we need from a carnot cycle between that sphere’s center and its exterior .
It saddens me that this is yet another non-science blog . I had hopes .
Good Bye .
Is it too late in the day to say good riddance?..;)
I’m probably just echoing what Weinstein is saying (sorry if I misunderstood you wrong), but I think the point is that Surface heat loss due to radiative processes is so minute at this level of optical depths, that it is really insignificant (in practical terms, no heat is lost due to radiation from the surface of Venus). According to my really simple model backradiation (another quite inappropriate term in my exceptionally humble opinion) multiplies the received surface radiation by (n+1) opaqe layers. Which would mean, that if the atmosphere absorbed 100% of radiation in 1 cm, the atmosphere of Venus would have to be 16100/170=95, 95-1=94 cm thick (in other words, less than a meter) to achieve the desired result through radiative processes only (this number seems a bit extreme, I’m guessing I made a miscalculation somewhere). Or to put it in other terms, we really don’t particularly care how much the surface of Venus radiates.
We must remember that only our planet as a whole faces the unfortunate condition of having only radiative processes as a way to lose heat. Any particular part of the planet itself doesn’t have that problem, as it can share it’s energy through other methods with rest of it’s motherplanet.
Malt,
IMHO you and Leonard are right when you point out how opaque the atmosphere of Venus in parts of the IR spectrum.
I am used to calculations based on the absorption rates for the gamma ray spectrum. The standard method involves the concept of “tenth value layers” that will reduce the gamma flux by a factor of 10. Then one provides as many layers as necessary to bring the flux down to whatever level you need.
Applying the same idea to pure carbon dioxide at a pressure 90 bars, the tenth value layer at 15 microns appears to be less than 1 mm. One needs to look at the rest of the relevant IR spectrum but I would be surprised if even 1% of the total surface radiation can escape directly.
Mait,
I am glad to see someone sees my point.
DeWitt,
The radiation heat transfer is very small (see my last post and Mait). The convection is far and away the main term in most of the atmosphere. The greenhouse gas only sets the height of the effective location of radiation to space. The dry convective lapse rate is the valid rate up to the upper troposphere. To give another analogy consider a hot radiating surface with a thick and good insulated cover held just above the surface, and a vacuum between them. The part of the insulation near the surface will receive radiation and heat up to near the temperature of the hot wall. A small amount of energy will be conducted through the insulation. If the insulation is good, the transmitted energy will be FAR less than the direct radiated energy. The reason is the hot underside of the insulation will radiate almost as much back to the wall as the wall radiates, and it is the difference that determines net heat transfer rates. The heat transfer for that case will depend only on the amount conducted by the insulation, even though the wall is radiating a lot. For that case, conduction rather than convection is the main player. For the atmospheres, convection is the main player, since the conductivity is so small.
Mike Blackadder:
You are right, I hadn’t thought about it clearly.
Leonard Weinstein:
Well put.
And I would like to see this analysis as well, I’ll see if I can find one.
I don’t think that this is Steve Goddard’s explanation. If Steve Goddard had provided your explanation and made this point I wouldn’t have written the article – unless I had an RTE solution which demonstrated that it was wrong. Well, the RTE is not solvable with a pocket calculator so I also might have questioned the validity of the solution if it was provided via some kind of “extrapolation”.
scienceofdoom,
I tried super-imposing the Jenkins, 1995 graph on top of the Fig. 4.3 from S. Manabe and R.T. Wetherald, 1967. Then I realised that Fig. 4.3 is not about Venus!
My understanding is that the graph shown in Jenkins, 1995 is measured data for Venus:

The lapse rate appears to be significantly less than the dry lapse rate (10.5 K/km), probably owing to sulphuric acid vapour condensing at heights above 30 km.
scienceofdoom:
At the top you wrote:
“If Venus had the same albedo as the earth, the energy absorbed per m² of surface area would be, E = 2,636 * (1-0.3) / 4 = 941 W/m².”
Isn’t 2,636 X (.7) / 4 equal to 461.3 instead of 941?
Bill Stoltzfus:
Thanks. Looks like even basic maths is beyond me. The post is now corrected.
gallopingcamel,
I wasn’t talking about conduction being dominant in the Earth’s atmosphere as it exists now, but in a hypothetical perfectly transparent atmosphere. Conduction is (correctly) ignored in the Earth’s atmosphere except at the surface where temperature gradients can be large enough that significant conduction can occur ( fresh black asphalt or dry desert sand on a sunny day for example).
If you can find a copy of A Climate Modelling Primer by Kendal McGuffie & Ann Henderson-Sellers, I suggest you read Chapter 4 on Intermediate Complexity Models. Here are some relevant quotes:
“The greenhouse absorbers not only affect the surface temperature, they also modify the atmospheric temperature by their absorption and emission of radiation”
“Radiative [only] equilibrium profiles [of temperature vs. altitude] are unstable to vertical (moist and dry) convection. Thus the tendency of radiative heating alone to produce large lapse rates adjacent to the Earth’s surface, as shown in Figure 4.9, is offset by a rapid vertical transfer of heat.”
In other words, radiation forces convection, as I stated above. The balance of radiation and convection results in a positive lapse rate. This is true whether you’re talking about a stellar or planetary atmosphere. The heat input and atmospheric radiative characteristics as well as the heat capacity and gravitational acceleration determine the surface temperature and lapse rate. The lapse rate does not determine the surface temperature just like the volume of the atmosphere does not determine the temperature, it’s the other way around.
It doesn’t matter that if you know the pressure and volume you can calculate the temperature. You can’t change the volume of the of the atmosphere unless you change the temperature. There are no cylinders and pistons surrounding the atmosphere.
The devil is always in the details. My issue is that all these calculations based on “global averages” simply don’t work for individual locations/situations. Thus, there is no empirical evidence for the conclusions.
I just happened to realize that I actually DO have a way to estimate backradiation at any given loOthcation at any given time. The MINIMUM ESTIMATE is the amount of radiation that corresponds to the night-time temperature. SO, if we have a night-time temperature of 25 C in Atlanta, we have the equivalent of a blackbody radiating at 447 wm-2. Now, at noon, on a clear July 4 we can add the solar insolation, about 800 wm-2, to that value, giving 1,247 wm-2, which is equivalent to 112 C (234 F). Of course, the actual backradiation at the hottest part of the day is even higher!
It doesn’t happen, folks. Otherwise, we could have steam turbines instead of mere solar cells.
You can get a crude estimate of the back radiation at night by using an IR thermometer (~$50 at your local auto parts or kitchen supply store). I just went outside here and the ground temperature was 25 C (298 K) with a sky temperature of -1.8 C (271 K) so we have 447 up and 306 down. The ground temperature should be reasonably accurate but the sky temperature (clear sky) is probably an underestimate. The difference between up and down should be closer to 90 W/m2. That would be 280 K or about 7 C. Still not bad for such a simple instrument. It would be pretty accurate for a cloud covered sky though.
Even though peak insolation is ~1000 W/m2, the heat capacity of the ground and the air above it means that you won’t get anywhere near the zero heat capacity black body temperature before the sun goes down. In the summer in the NH at mid-latitudes, the phase shift of peak temperature is about 6 hours or 90 degrees, which means the time constant for temperature is an order of magnitude or two longer than a day.
DeWitt:
Yeah, but…it seems to me that you could put a piece of black foil on styrofoam to get a surface with essentially zero heat capacity. Cover the whole thing with polypropylene and measure the temperature. Probably never gets anywhere near 112 C (I guess I need to try this). A greenhouse does not have much of a lag time, so I don’t think you are correct about long lag times, unless you are talking about something that is fairly transparent, like a tank of water–or something with very high conductivity, like copper.
Regardless…my whole point has always been exactly what you say–that there IS, INDEED, a very large heat capacity provided by the surface and the atmpsphere. BUT the heat that is stored is not being “considered,” or “counted” when only radiation cartoons are being used in an attempt to explain temperature regimes!
DeWitt says this:
“As I said above, the atmosphere has a near adiabatic temperature profile because radiative heat loss drives it to that profile. In the absence of absorption/radiation by the atmosphere, conduction would dominate and the atmosphere would be nearly isothermal.”
DeWitt, doesn’t near surface heating proceed by, in the CO2 relevant wavelengths, collisional distribution of absorbed radiation? This collisional transfer creates LTEs which are then convectively carried vertically to an emission layer where an isothermal balance between the LTE and the surrounding air is achieved by the convection process. At the emission layer radiative process of the LTE air resumes. Is that what you mean?
JAE says:
The averages are derived from many different values in many different locations.
So for others reading this who are new to the subject this (downward longwave radiation) is a parameter that has been measured in many places at many times. Unfortunately, it is not as ubiquitous as temperature measurements because the equipment is a lot more expensive.
Downward surface radiation is monitored continuously by the Baseline Surface Radiation Network around the globe. And of course innumerable studies in meteorology and atmospheric physics have also measured it.
Downward radiation from the atmosphere is not particularly mysterious, or difficult to understand, or difficult to measure.
But for people uncomfortable with the inappropriately-named “greenhouse” effect, downward radiation from the atmosphere is something which causes much confusion. I expect to do another post fairly soon just to bring all of common questions into one place.
DeWitt,
This is what I like about this site. One can learn a bunch of interesting stuff without any derision or name calling. As usual you have illuminated the subject under discussion in some depth.
However you still have not addressed my original criticism of one of your earlier statements (June 13th). Here is what you said:
“In the absence of radiation absorption/emission by the atmosphere, the lapse rate would be very small. ”
This is flat out wrong because convection gets the job done at least in the troposphere.
Later on you contradict yourself by saying:
“But in the real atmosphere, any increase in the lapse rate above the adiabatic rate causes convection to make up the difference.”
While you and Chris Colose clearly have much greater knowledge of the subject than most of us that does not make you immune to errors or careless statements.
I don’t see an inconsistency in those statements. Vertical convection causes the lapse rate to decrease always. You’re moving heat from a warm surface to colder upper atmosphere and reducing the temperature difference. There’s no way that I can think of for convection to increase the lapse rate. It’s probably a Second Law violation. Any lapse rate less than the adiabatic rate, however, is at least conditionally stable so there is no driving force for convection. But if there’s a temperature gradient, there will be heat flow. Since heat flows from warmer to colder, this heat flow will act to reduce the temperature gradient over time and the transparent atmosphere will become even more stable. In the Earth’s atmosphere, however, radiation to space is a heat loss that acts to constantly make the atmosphere unstable and forces convection to reduce the lapse rate to the adiabatic rate, to a first order anyway.
The meridional temperature gradient for a spherical planet exposed to the sun on one side complicates matters as it causes horizontal heat movement or advection. This would happen even for a transparent atmosphere. The cause is what’s called the pressure gradient force. At the poles, the altitude for any given pressure is lower than at the equator. So at constant altitude, there’s a pressure difference between the equator and the poles that increases with altitude. If the planet didn’t rotate, the flows would be fairly simple, but it does and they aren’t. One of the results are the jet streams in the NH and SH.
Admittedly, I don’t put much trust in “energy balance” diagrams having seen examples from the early 1970s where the totals was just as “plausible”, but there was no reference to the greenhouse effect.
This is why I try to start from the very basics. For example,a couple of “gedanken” experiments:
(a) if Earth were to experience a massive gas exchange episode, including a complete removal of all greenhouse gases from the atmosphere and an increase of atmospheric mass so that the surface pressure would be as on Venus at the moment, what would the terrestrial surface temperature be?
(b) alternatively, if Venus were to lose 89% of its atmosphere tomorrow, with the remaining bit becoming more opaque so that the amount of solar radiation hitting the surface wouldn’t change, what would the Cytherean surface temperature be?
Jae,
“t seems to me that you could put a piece of black foil on styrofoam to get a surface with essentially zero heat capacity. Cover the whole thing with polypropylene and measure the temperature.”
What you’re describing is a simple pyrheliometer. Here’s a link to the description of a commercial instrument: http://www.eppleylab.com/PrdNormIncPyrhelmtr.htm
It’s certified to be linear to 1400 W/m2.
DeWitt,
The radiation heat transfer near the surface of Venus is very small. Almost all of the heat transfer is convective. For the case of Venus, convection does not increase (or decrease) the lapse rate, it causes it. If the pressure is changing at the same time the temperature is, as is the case for increasing altitude, there is NO conduction heat transfer, even though there is a temperature gradient. This is called adiabatic flow (the work done by expanding as gas rises to increased altitude cancels the excess temperature from energy being transported). This would be even true for diffusion rather than convection of molecules to higher altitudes. The opposite occurs as gas drops. The increased pressure raises the temperature. This is basically a trade off of gravitational potential energy for thermal kinetic energy.
You still have cause and effect reversed. Convection does not cause the lapse rate. A lapse rate in excess of the adiabatic rate causes convection. The magnitude of the adiabatic lapse rate is explained by thermodynamics. But the actual lapse rate is determined by the balance between radiation and convection with the latent heat of water vapor playing a very large role.
In the absence of convection there would still be diffusion. Diffusion happens regularly on clear, calm and cold nights when a temperature inversion near the surface occurs. It happens on a larger scale in the Arctic winter. The temperature profile varies with altitude and time exactly as expected for diffusive heat transfer from warm air to a cold surface. That’s the basis, btw, for the Klotzbach, et. al. paper on trend exaggeration for 2 m mounted thermometers. ( http://www.climatesci.org/publications/pdf/R-345.pdf , the link doesn’t seem to be working right now ).
If the atmosphere below the cloud layer of Venus were transparent, it would have to be isothermal. If it were not, there would be an excess or deficit of radiation to space at the cloud layer because the surface would be warmer or colder than the cloud layer..
Sounds more like incorrect terminology than being confused about cause and effect.
As mentioned by Weinstein, the adiabatic lapse rate follows from conversion between potential and kinetic energy and does not involve an exchange of heat (ie. isentropic). Unless I’ve been led astray by Wikipedia (which is possible), then this does ultimately follow from PV = nRT and T2 = T1 x (p2/p1)^[(Gamma-1)/Gamma] where Gamma is the heat capacity ratio of the gas – for isentropic process.
So the lapse rate is visualized as following the same process as convection where a higher temperature body of gas under higher pressure can move higher in the atmosphere and occupy a larger volume at a lower temperature and pressure. The difference being that for the adiabatic lapse rate that no heat is transferred in the process. Or perhaps you could say the higher temperature gas is prevented from expanding by the higher pressure surrounding it, and only when the temperature exceeds the adiabatic equilibrium that convection occurs and heat is actually transferred.
Omnologos,
a) If ALL greenhouse gases were removed, the surface of Earth would be about 33 degrees C cooler, even with a much denser atmosphere. However, if even a fairly small amount of greenhouse gas were present (and that includes water vapor), and the quantity of other gas increased to near Venus level, the temperature would rise several hundred degrees C.
b) Removing a large mass of Venus’s atmosphere would lower the surface temperature considerably, even if the remaining gas were passing the same amount of input energy from the Sun.
DeWitt:
“What you’re describing is a simple pyrheliometer. Here’s a link to the description of a commercial instrument: ”
Thanks for the link. Aren’t the pyrheliometers normally used to measure solar insolation? If so, they need to block long-wave IR. Hence the “filters” that are described??
I want to see how the solar insolation compares to the total radiation, including the “backradiation.”
If you clicked on the Eplab link at the bottom of the page you would have found their other instruments including this one: PRECISION INFRARED RADIOMETER ( http://www.eppleylab.com/FrmInstrumentation.htm )
“The Precision Infrared Radiometer, Pyrgeometer, is intended for unidirectional operation in the measurement, separately, of incoming or outgoing terrestrial radiation as distinct from net long-wave flux. The PIR comprises a circular multi-junction wire-wound Eppley thermopile which has the ability to withstand severe mechanical vibration and shock. Its receiver is coated with Parson’s black lacquer (non-wavelength selective absorption). Temperature compensation of detector response is incorporated. Radiation emitted by the detector in its corresponding orientation is automatically compensated, eliminating that portion of the signal. A battery voltage, precisely controlled by a thermistor which senses detector temperature continuously, is introduced into the principle electrical circuit.
Isolation of long-wave radiation from solar short-wave radiation in daytime is accomplished by using a silicone dome. The inner surface of this hemisphere has a vacuum-deposited interference filter with a transmission range of approximately 3.5 to 50 µm.”
Thanks. Now, where is the data from both the pyrgeometer and the pyrheliometer for noon at a given location? I can get the daily averages here:
http://mynasadata.larc.nasa.gov/las/servlets/dataset?catitem=21
But I haven’t figured how to get hourly averages.
“- An internal heat source into the surface of Venus is one solution to the problem.”
“- The “super greenhouse” effect from a highly opaque atmosphere of CO2 and a little water vapor is another solution.”
“supernatural forces is another”
Question this:
How does it come to pass that the absurd explanations are given equal time and equal billing with the ONLY common sense one?
Somebody has a a bete noir – maybe charcoal – and this eminence grise – probably C02 – is the first fetish invoked as the universal answer to every darn question- even completely unrelated ones. Very catholic.
Look at Venus- it’s repaved by lava recently and frequently. The liquid rock is just below the surface- look at the type of volcanoes.
The ground is hot because it’s a thin skin floating on magma, duh. It doesn’t cool fast because it’s got a really crappy heat pump- due to lack of a phase change in the working fluid.
It’s not because somebody skipped prayers or cuz of that devil CO2. Goddard’s silliness was CO2 fetishism, nothing more, as is this.
(Disclaimer- the co2 made me write this – held me down, beat me with a boltzman and blacked my body – wasn’t my idea… do you believe that one? Other people it makes them write bad checks. Still others it makes them write volumes of bad prose.)
But Dave, don’t you know 160W/m2 reaches the surface, about the same leaves the TOA but the surface radiates 16000W/m2, so it must be greenhouse:-)
Dave McK:
As Nick Stokes already pointed out, if this is the source of heat and there is little absorption of IR, then the measured radiation out to space would be much higher than the sub 200W/m^2.
The surface is radiating 16,000W/m^2.
So volcanic activity is a plausibe source of heat, except the measurements we have taken of the outgoing radiation don’t back up this theory.
DeWitt,
You can’t have an atmosphere below the clouds optically clear, but above absorbing, as the gas is the same. If the clouds were the only long and short wave absorbers (in a totally non-absorbing gas), the clouds would be the location setting the temperature, but there would still be a lapse rate both below and above, with the temperature going down above and up below.
I do not understand why you keep saying that I claim the convection increases the lapse rate. It causes it, and the level is almost exactly the calculated value of g/Cp.
For the actual case of Venus, the different Solar angles at different latitudes cause Hadley cell circulation and the planet rotation (and thus temporal variation of Solar insolation) causes more circulation. These are the main drivers for the convection. If there were no convection, then diffusion and radiation would be the source of the lapse rate, but for Venus, both of these are small compared to convection. The lapse rate plus the location of the actual radiation out are the cause of the hot surface.
It’s a thought experiment. If the atmosphere were transparent, then there would be no convective heat transfer and a lapse rate of zero.
Look at it another way. If have a model of the Venusian atmosphere and initialize it as isothermal at the surface temperature of 730 K, what happens? The top of the atmosphere cools by radiation because it’s radiating at twice the rate of absorption. That propagates downwards until the lapse rate reaches the adiabatic rate. Then we start to see convection because the radiative only lapse rate exceeds the adiabatic rate. Similarly if we initialize the model with an atmosphere at 230 K below the cloud layer and a surface temperature of 730 K, the atmosphere will be warmed by convection and radiation until the lapse rate reaches the adiabatic rate. At that point, radiation and convection are in balance.
“If the atmosphere were transparent, then there would be no convective heat transfer and a lapse rate of zero.”
OK, so a Diesel engine wouldn’t work in, say, a pure nitrogen-oxygen atmosphere?
Craig Goodrich
Yes a combustion engine would still work, but you are adding heat/pressure chemically, to an enclosed area, and harnessing the expansion, this is where you are drawing your energy from in an engine. It has nothing to do with radiative absorption of SW or LW…
SOD,
There may be some internal source of heat on Venus, but it is not likely to be significant based on the example of Earth, and what is known of the composition, size, and core of Venus. The surface is hot due to the lapse rate and some greenhouse gas and the high atmosphere mass. Nothing else is needed.
SOD,
Everyone keeps getting carried away by the fact that the surface is radiating 16,000 W/msq. However due to the superhigh optical density, including pressure broadening, the back radiation to the surface is also nearly 16,000 W/msq. The net radiation heat transfer is much less than 1 W/msq in almost all of the atmosphere. Convection totally dominates the heat transfer. Look at my example for the hot surface with an insulator above it. Even if all the incoming Solar radiation is absorbed before the surface (such as at the cloud layer), it would eventually get to the ground by conduction, convection and radiation in a non-equilibrium state until the present temperature distribution were established, so nothing special is needed to start the process. The fact of outgoing radiation being limited to the upper atmosphere sets that temperature location.
I for one am not carried away. The whole point is that the atmosphere of Venus is opaque. The argument that’s being countered by emphasizing the radiant flux at the surface is that the surface would be just as hot if the atmosphere weren’t opaque or was even less opaque.
If you replace some of the CO2 in the Venusian atmosphere with a transparent gas, then the opacity will decrease and the radiative thermal conductivity of the atmosphere will increase. The reason is that the mean free path of a photon will increase. Therefore the surface temperature will drop. But so will the cloud layer altitude because the average temperature of the atmosphere will also drop and the density increases. So the lapse rate should remain the same.
That should be diffusivity not conductivity. I keep making that mistake. This affects the saturation argument as well. Even if 99.99% of IR photons are absorbed in some relatively short distance, an increase in concentration will still shorten the mean free path of a photon and decrease the radiative diffusivity. The same thing happens with thermal diffusivity which is inversely proportional to density. So my argument that heat transfer by conduction is higher at the surface of Venus than the Earth is wrong. It’s lower.
DeWitt,
You are right that if most of the CO2 were replaced with an equally dense but optically transparent gas the the opacity would decrease. However, even for a drop of 99 percent, the remaining CO2 would still be 2300 times the Earth level. This is still optically opaque to outgoing radiation, and the resulting temperature change only a few degrees. It is not the cloud layer that controls most of the outgoing long wave radiation, but the CO2 well above that. The cloud layer absorbs and partially reflects the shorter wave Sunlight, so that is what we see.
“However, even for a drop of 99 percent, the remaining CO2 would still be 2300 times the Earth level. This is still optically opaque to outgoing radiation, and the resulting temperature change only a few degrees.”
Bullock and Grinspoon, among others, would beg to differ. See Figure 2. here: http://www.boulder.swri.edu/~bullock/Homedocs/GSRP94.pdf
According to them, even a small decrease in CO2 would lead to a collapse to a much cooler planet by absorption of CO2 by the crust. If there’s more CO2 left in the crust (which seems unlikely) Venus could potentially get a lot hotter.
Hashimoto and Abe ( http://www.terrapub.co.jp/journals/EPS/pdf/5203/52030197.pdf ), OTOH, think that there’s an albedo feedback mechanism that maintains the surface temperature for variation in solar flux and CO2 concentration, down to 30 bar surface pressure anyway.
In other words, you can always get more opaque as there’s no such thing as perfect opacity (or transparency or reflectivity for that matter).
Science of Doom,
If you are going to calculate a case of less CO2 (replaced with Argon), please use replacement of 99 percent rather than 99.99 percent. I think I may have pushed too far based on the altitudes of matching the Earth partial CO2 pressure for radiation out. I looked at the lowering of altitude where the partial pressure matches Earths CO2 partial pressure at Earths tropopause and the drop for Venus was from 90 km to about 60 km for 10,000 X drop, but only went from 90 km to about 84 km for 100 X drop. That along with the lapse rate and troposphere temperature would be closer to my guess of just a few ten’s of degrees change. The 10,000 X drop in CO2 would still result in a very hot surface, but would probably be over 100 degrees less hot, due to large drop in tropopause level, and similar lapse rate.
DeWitt,
The only issue I am discussing is why is the surface hot at the present, and what would happen (for no surface chemistry change) if the atmospheric composition were different.
I don’t quite understand why gravity can’t cause a temperature gradiant in a atmosphere (and hence a higher surface temperature). I you set out an air molecule on a free path upwards from the surface at 500 m/s, it would fly upwards for a while and then start falling down again (gravitational acceleration). If you measured the speed of the molecule at different hights, you would get quite a clear difference in speed vs height. This ought to translate into a temperature gradient due to the relationship between kinetic energy and temperature (or more precisely, I can’t figure out why it wouldn’t).
Basically, that’s how the adiabatic lapse rate is calculated. The adiabatic lapse rate is the trade-off between kinetic energy and gravitational potential energy. Take a kilogram of dry air at a temperature of 30 C at the surface. The heat capacity at constant pressure of dry air is 1004 J/kg/K so the heat content relative to 0 C is 30120 J and the gravitational potential energy is zero. If we raise that kilogram 1 km against the acceleration of gravity then we have done work equal to the acceleration of gravity times the distance moved or 9810 J. If we remove that amount of kinetic energy from the kg of air, it will then have 20310 J, which means a temperature of 20.23 C, so the dry adiabatic (meaning no exchange of energy with the surroundings) lapse rate is 9.77 K/km.
So when you move air at the adiabatic lapse rate up or down, it stays where it is because there’s no difference in temperature between the air and it’s new surroundings. If the lapse rate is greater than the adiabatic rate and you move air up or down it will be warmer than the air around it and will want to rise and keep rising. That’s unstable. But if the lapse rate is less than the adiabatic rate, if you move the air up, it will be colder than the air around it and want to fall back down. Move it down and it will be warmer and want to rise back up, but no higher than where it started. That’s stable.
But if you restrict all horizontal motion, you still have conduction, which is basically diffusion. Things diffuse along gradients, or a change of a property with distance. Trace gases diffuse all over the atmosphere. That’s how CFC’s get into the stratosphere to affect the ozone concentration. Temperature behaves the same way. Heat flows along a gradient to reduce the magnitude of the gradient. It doesn’t matter if the gradient is vertical or horizontal. In the absence of radiation, once heat gets into the upper atmosphere, it stays there and a one dimensional column would eventually become isothermal. In the real atmosphere, though, radiative and convective heat transfer are many times larger than conduction so it is normally ignored except near the surface where the temperature gradient can get quite large either for cooling or heating.
De Witt, I presume you have seen this:
http://landshape.org/enm/on-the-use-of-the-virial-theorem-by-miskolczi/
This is from the linked analysis by Giger:
“the temperature (the KE) has to be
measured with a thermometer, whereas Eu represents the radiative temperature (flux) that has to
be measured with a radiometer, and these two measurements can give vastly different results as
we see for the two following extreme cases:
The first case is a totally transparent and non-absorbing, and therefore non radiating IR
atmosphere, where Eu=0. This case may be approximated by an atmosphere consisting of O2
and N2 only, with CO2 and water vapor absent. The VT holds in this case as it always holds for
any gas, but the kinetic energy (temperature of the gas) is not visible as thermal radiation since
O2 and N2 don’t radiate in the IR region (Eu=0). Again, we have to measure the temperature of
Eu with a radiometer and not a thermometer (convection)! Fig.1 would simplify to: OLR=St=Su,
Eu=Ed=0 and no GH effect would exist, the Earth’s surface would be about 30 deg C cooler and
the O2-N2 atmosphere would further cool as usual with altitude according to the dry adiabatic
lapse rate.
In the second case we have the completely opaque thick atmosphere (saturated with GH gases
and water vapor), where no IR flux (St=0) propagates from the surface (Su) into space and
OLR=Eu. Since only the top of the atmosphere radiates into space it would not be representative
of the much higher average temperature of the thick atmospheric layer or its KE. We actually
have a Venus like atmosphere with Ts (surface temperature) much larger than Te (greybody
temperature) where Su>>OLR=Eu.”
cohenite,
It’s a mistake to think that any real gas is perfectly transparent. Even for the noble gases, there’s still collision induced absorption/emission. In addition, oxygen has a permanent magnetic dipole so it has lots of rotational lines in the microwave region of the spectrum. 60 GHz should ring a bell as that’s the frequency used by the AMSU in satellites for measuring atmospheric temperature profiles.
For the rest, I second Nick Stokes: “The graphs are arm-waving with a pencil.”
If say a 4um IR wavelength leaves the planet surface it has 8 to 10 times the translational KE of any CO2 molecule it meets.(E=hf)
All CO2 molecules in the photons path have the potential to absorb this photon.
Now by the equipartition of energy the extra KE absorbed by such a co2 molecule is quickly shared about with the other molecules given that 10 to the power of ten collisions this molecule experiences.
How likely is it that a Co2 molecule will have 8 times the KE of its neighbours?
However to re-emit a similar wavelength requires the same molecule to achieve 8 t0 10 times the average KE of a typical air molecule at that temperature.
The re -emission is statistically highly unlikely!
There seems to be an asymmetry between absorption and emission!
Those readers who are familiar with statistical mechanics may have an answer to what seems to me to be a paradox where Kirchoff’s Law seems to be violated.
You’re confusing emissivity with emission. There is no requirement that the same number of photons are emitted at a given wavelength as are absorbed. It certainly isn’t true at the Earth’s surface or a for blackbody with a brightness temperature less than the emitting body, say 300 K vs 6,000 K, so why should it be true for CO2? The emissivity of CO2 at 4 micrometers could be 1 and there still wouldn’t be much emission at 4 micrometers because you multiply the emissivity at a wavelength by the Planck function for the temperature and wavelength to get emission and the value of the Planck function at 4 micrometers or 2500 cm-1 is small at 300 K. Absorption is only a function of concentration, path length and absorptivity. Molecular absorptivity has some dependence on temperature because of line broadening, but it’s a much smaller effect.
Goddard and Motl are analyzing Venus and Earth from a “convection-centric” perspective rather than SOD’s “radiation-centric” perspective. Either perspective should be able to produce the correct answer. On both planets, buoyancy considerations and the ideal gas law predict that convection efficiently transfers heat absorbed by the surface into the upper atmosphere whenever the temperature gradient with altitude (lapse rate) is steeper than a critical value determined by the ratio of local gravity to the specific heat capacity of the atmosphere. For Venus, this appears to be about -9 degK/km, while for earth the value is about -6.5 degK/km (different because of latent heat from condensing water vapor). In the case of the earth, once energy has risen about 7(?) km by means of convection, the concentration of GHG’s is low enough that outgoing radiation is equal to incoming solar radiation adjusted for albedo. Therefore, the surface of the earth is about 45 degK warmer than the temperature at the altitude where incoming and outgoing radiation are in equilibrium. On Venus, energy needs to rise about 60 km by convection before it can escape as OLR through the remaining GHG’s in the Venusian atmosphere. This makes the surface of Venus about 540 degK warmer than the altitude where incoming and outgoing radiation are in equilibrium.
So who is right, SOD or Motl? (As Motl points out, Goddard’s analysis (as usual) isn’t properly grounded in fundamental physics, so the question really comes down to SOD vs. Motl.) And SOD, as usual, appears to be fixated on radiative transfer – in this case the 16,100 W/m² leaving Venus’s 700+ degK surface. However, it shouldn’t make any difference whether the surface of Venus radiates 1,610, 16,100, or 161,000 W/m² – the fixed slope in the OBSERVED temperature vs. altitude graph tells us that convection, not radiation, controls energy transfer in most of the Venusian atmosphere. (See Figure 2.9 in CO2 – An Insignificant Trace Gas? – Part 3. Notice that radiative transfer leads to a curved plot, while convection produces a linear plot. Radiative transfer produces a curved plot because the amount (partial pressure) of a GHG drops exponentially with altitude, as shown in SOD’s post on Tropospheric Basics.)
If 90% of Venus’s carbon dioxide were replaced by nitrogen, the altitude at which radiative cooling takes over from convective cooling will be lower. On earth, pressure drops 10-fold in the first 17 km, but CO2 (44) is denser than nitrogen (28), so the drop in pressure with altitude will be more rapid and take place over perhaps 10 km. So radiative cooling from a Venusian atmosphere with 10% CO2 will become effective about 10 km lower in the atmosphere, translating (through lapse rate) to a surface 90 degK cooler. If CO2 drops to 1% – 180 degK cooler; 0.1% – 270 degK cooler; 0.01% – 360 degK cooler; 0.001% – 450 degK cooler; 0.0001% – 540 degK cooler. Since CO2 on earth is 0.0004%, we might expect about 500 degK cooler – which is in the ballpark for being correct. This agreement is fortuitous because I’ve ignored the following problems: a) Venus’s surface pressure is 100 times Earth’s. b) The optical properties of carbon dioxide change dramatically with pressure and temperature. c) The rate of change in pressure with altitude changes as carbon dioxide is replace by nitrogen. d) ?. Still, replacing 90% of the carbon dioxide on Venus with nitrogen is likely to reduce the surface temperature about 100 degK, somewhat more than the “few tens” of degrees that Goddard and Motl estimate.
So how can a “convection-centric” skeptic answer SOD’s challenge: “But for those who believe that high Venusian atmospheric pressure and the ideal gas laws cause the high 730K surface temperature – they have to explain how the heat is transferred to the surface so that it can radiate at 16,100 W/m².” The answer is fundamentally simple: Even a small amount of solar radiation will continue to warm the surface of Venus to higher and higher temperature as long as the rate of energy loss from the surface by convection and NET “long wavelength” (non-solar) radiation is smaller. As we have seen, buoyancy-driven convection doesn’t begin to remove energy from the surface until the lapse rate is about 9 degK/km and the surface temperature is 700+ degK. Why didn’t the 1453 W/m² of upward radiation from a 400 degK Venusian surface stop the surface temperature from rising? Or 3544 W/m² from a 500 degK surface? Or 7348 W/m² from a 600 degK surface? The answer appears relatively simple: The downward flux of radiation from the Venusian atmosphere is nearly identical to upward flux leaving the surface by radiation. Interestingly, the post cited above by SOD (bartonpaullevenson.com/Saturation.html) demonstrates why this is so. If we look at Table 1, we see that only 1/e of radiation at 13-17 um penetrates 1.48 meters through 1 atm of carbon dioxide – which I’ll round to 50% absorption for every 1 m. With 100 atmospheres of carbon dioxide on Venus, 50% of the radiation at 13-17 uM is absorbed in the first 1 cm of atmosphere. A lapse rate of about 10 degK/km means the temperature 1 cm above the surface is only 0.0001 degK cooler than the surface. Since the atmosphere and the surface that are in radiative equilibrium with each other have the same temperature, the NET energy flux in the upward direction is zero!. Of course, radiative energy transfer takes place through wavelengths besides 13-17 um. Looking at other wavelengths in Table 1, we see that the weakest absorption band for CO2 (1.9-2.1 um) absorbs 50% of radiated energy when passing through less than 5 m of CO2 at 100 atm pressure. And the temperature difference between the surface and 5 m could be too small for effective radiative cooling. What about the “windows” where CO2 doesn’t absorb outgoing OLR? Barton provides us absorption data for CO2 on earth: <1 atm total pressure and <300 degC. The absorption lines for CO2 are substantially broadened by the much higher pressure and temperature of the Venusian atmosphere. The absorption due to C13 (1%), O18 (0.2%), and 017 (0.04%) isotopes of carbon dioxide are important in an atmosphere which contains more than 100,000 times as much CO2 as the earth's. When the surface pressure is 100 atmospheres, the absorption due to minor GHG's becomes more important. If the average photon of long wavelength radiation near the surface of Venus travels 10 m vertically before being absorbed, the temperature difference will be 0.1 degK. At 730 degK, this will produce 16,102 W/m² flowing from warmer to cooler and 16,903 W/m² flowing from cooler to warmer – a net flux of only 9 W/m²! In theory, if only 10 W/m² of solar radiation penetrates to the surface of Venus, the planet could warm up to 700+ degK – at which point buoyancy-driven convection is able to lift energy from the surface to the upper atmosphere where it can escape to space.
Convection and radiation are linked. Ask yourself why convection continues when the adiabatic lapse rate is achieved. The atmosphere is conditionally stable at that point and there is no driving force for convection from differences in buoyancy with altitude. The reason is that radiation in the absence of convection would create an unstable lapse rate greater than the adiabatic rate. For the relatively thin atmosphere of the Earth, convection only needs to carry ~60% of the heat from the surface. In the optically thick atmosphere of Venus, the percentage is much higher, close to 100%. If radiation weren’t being absorbed and emitted at every level of the atmosphere, there would be very little convection.
Frank,
You are correct that convection and not radiation is the main heat transfer cause. However you have a few minor errors. The lapse rate of Venus is about -7.7 K/km, not -9 K/km. Next, you would have to use a non absorbing gas of near equal density as CO2 to replace it to maintain the pressure profile. Argon is a fair choice. If you drop the partial pressure of CO2 density 90 percent, the altitude of radiation to space would drop almost 10 km, but due to the more gradual drop off rate (due to the much thicker atmosphere), the temperature may not be quite as much as the nearly 77 K less warm. However, it could be more that 10 to 30 K less, so the point of Lubos Motl, Weinstein, and Goddard may not be real close to the actual difference, but their point is mainly that it is not necessary to have nearly as much greenhouse gas as stated to have a hot surface, and much more greenhouse gas only has a relatively small additional effect.
Where does the Venusian lapse rate come from? Shouldn’t the lapse rate for Venus be higher than earth due to heavier (heavier molecules) atmosphere? Gravity is lower as well, but from my very basic calculations the weight difference should be bigger than the gravitational diffence.
Mait,
It is specific heat, not molecular weight, that determines lapse rate. The mass enters in the pressure, since pressure is due to nothing but the weight of the amount of atmosphere above a given height.
Frank,
The g/Cp for Venus would indicate a value of lapse rate over -9 K/km. However, the measures value shown on the web shows -7.7 K/km from the surface to about 60 km, then dropping off. I don’t know if that is correct, but is shown on Wikipedia.
Ive got to ask… does it matter if no light reaches the surface, and SW or plasma (solar winds) heat the atmosphere from altitude?(say 50km) It would still surly (in time) end up with a similar temperature gradient… wouldn’t it?(it can still only leave from TOA… and not really showing anything similiar to our stratosphere…
What effect do the solar winds have on the upper atmosphere? Just something that those temp gradient charts got me curious about.
SOD,
Here is a simple thought experiment: Consider Venus with it’s existing atmosphere, and put a totally opaque enclosure (to incoming Solar radiation) around the entire planet at the average location of present outgoing long wave radiation. Use a surface with the same albedo as present Venus for the enclosure. What would happen to the planetary surface temperature over a reasonably long time? For this case, NO Solar incoming radiation reaches the surface. I contend that the surface temperature will be about the same as present. Now assume the gas temperature and distribution are initially different from present levels, but no gases are condensed other than those that form the present clouds. I contend the temperature will go to about the present distribution and level. The only reason for this not to not match exactly the same as the actual atmosphere would be due to the fact that for the atmosphere, outgoing radiation actually leaves from a finite gas thickness, but the present version is a surface.
OK, I’ll bite. I disagree. A lapse rate needs energy to maintain it as non-zero, otherwise conduction will take it to uniform temp.
On Earth, and Venus, the free energy comes from an entropy differential. Heat from solar is delivered at relatively high temp at the surface, and sent back to space at low temp. The heat flux moving from high to low temp is able to drive various heat engines. These create the atmospheric motions that, through the adiabatic mechanism, maintain the lapse rate.
There are frictional losses etc that create entropy. This entropy has to be transferred to space, for steady state. Again this is achieved by absorbing solar at a hot surface and emitting from a cold.
But with your shell, there is no differential. Incoming solar is absorbed and emitted at the same temperature. There is no free energy to create motion, maintain temp gradients etc.
SOD,
One point I left out, assume the surface has a black body emissivity for the long wave outgoing radiation.
SOD,
Also assume it is a thin but good conducting material.
Leonard Weinstein:
Let’s call this enclosure “E”. And the surface “S”.
E receives 158 W/m^2 (absorbed solar radiation).
E radiates as a blackbody out to space “has a black body emissivity for the long wave outgoing radiation“.
Does E radiate as a blackbody downwards to the surface? Critical piece of information
E is a “good” conductor through to the gaseous mixture below.
E is “thin” so this implies a low heat capacity and an isothermal (no temperature gradient) layer
One way to solve the problem is to assume that E & S start off very cold and see what happens.
E receives 158 W/m^2, and heats up quickly.
Scenario 1:
Heat loss per second from E:
– by radiation to space – sigma x T^4
– by radiation to the surface – 0
– by conduction to the surface – low (see note)
Therefore, this layer quickly heats up until T= 230K (158/sigma)^0.25 when it radiates at 158 W/m^2.
And very low heat is conducted to the surface.
Scenario 2:
Heat loss per second from E:
– by radiation to space – sigma x T^4
– by radiation to the surface – sigma x T^4
– by conduction to the surface – low (see note)
Therefore, this layer quickly heats up until T= 193K when it radiates at 158 W/m^2 (both directions at 79 W/m^2 each).
And very low heat is conducted to the surface.
Note: the layer is very conductive but the gases are not conductive, therefore although the top layer of the gases heat up (according to heat capacity) this can’t induce convection as only the heated top layer will “rise”.
In scenario 1, the surface and atmosphere will not heat up.
In scenario 2, the surface will heat up from the 79 W/m^2 directed at it.
Before going any further, is this the correct description of the system? Did I understand it correctly?
Leonard Weinstein
I have re-read Steve Goddard’s article 3 times and still don’t know what he really thinks. I read most of his comment responses and am still unclear.
I don’t want to be a “quote miner” to try and establish something the person doesn’t believe, so if Steve Goddard is reading perhaps he can explain..
Here are some extracts of his responses to comments on his article:
I don’t take from this that Steve Goddard believes that a high “greenhouse” effect is in existence and that convection is initiated from this large “greenhouse” effect.
I read from this, that pressure causes temperature and the “greenhouse” effect, while in existence, is not really that important. It doesn’t seem to be the driver for the high surface temperature of Venus (in SG’s view).
I welcome Steve Goddard explaining his point of view in a little more detail here. And I apologize in advance if I have misunderstood his article and follow up comments.
Leonard Weinstein:
following my comment on Goddard/Motl
And for Lubos Motl, the same applies, perhaps I have misunderstood his article, he is welcome to explain his views more specifically. Here is his conclusion:
The first paragraph says (to me) that Motl doesn’t understand the “greenhouse” effect. And the second paragraph on its own might be a good explanation – but in the light of the first paragraph..
First paragraph – “the lapse rate is a pre-requisite for the greenhouse effect”? “Without a lapse rate absorption and emission would be balanced” – true.
But with it, absorption and emission would also be balanced.
My comment – without the lapse rate the surface would be hotter.
And with the lapse rate the surface is cooler.
The back-radiation from the “greenhouse” effect creates the conditions for convection. This is well-explained by DeWitt Payne many times within the comments on this article.
Well, if Lubos Motl wants to explain his point of view, it would be very welcome, and I apologize in advance if I have misunderstood his article.
I do not wish to defend any other position than my own. I agree with Steve and Lubos on the net effect of the greenhouse gas, i.e., to move the location of the outgoing radiation up to the outer layer of the atmosphere rather than the surface, and the lapse rate (due to a gravity effect) would make the surface hotter. I can’t comment on their choice of words.
Regarding the case I gave above, the thin opaque layer would radiate equally well on both sides. The amount of radiation would be (on average) 158 W/msquare BOTH ways, and correspond to a so called average temperature of 230 degrees K. However, the temperature would vary a lot locally due to night and day and different latitudes. The variation along with downward longwave radiation would cause convection, diffusion, and radiation induced currents which would, over a period of time, reach the surface and set the lapse rate to near the present value. You might ask how the layer can radiate 2 times the Solar input, but that is no different than how the surface can be hot. There is a transient effect where the surface accumulates more energy than radiating, until it reaches the final balance. Some of this accumulating energy is distributed in the gas below and some is trapped. Eventually the gas below is sending 158 W/msquare back to the underside of the layer to balance the radiation from the layer, so no net energy is passed. Due to different temperatures at different locations, the actual fact would be that some areas take in more than send out, and some do the opposite. The average is balanced. The final result would be about the same surface temperature as present.
SOD,
You do not need a greenhouse effect to get convection. Radiation between gas layers is not the only (or generally the main) cause of convection. Local accumulation of surface energy on a planet, even for no greenhouse gas, would result in heat transfer to the gas above it, and thus induce a rising current. Variation at different locations (different latitudes and day/night differences) cause surface winds and 3-D motion (convection). Radiation to the gas is not needed. The main result of the greenhouse gas is just to raise the overall temperature by changing the location of radiation to space.
Leonard Weinstein
…”You do not need a greenhouse effect to get convection.”..
I am in total agreement.
There are thinks that AGW adherents and sceptics can disagree on but this is surely not one of them.
Carts and horses chicken and egg seems to spring to mind.
Air particles hitting the planet surface take on the characteristic temperature of the warmer surface.
Faster air particles leaving the surface will produce a higher pressure.
The warmer air pushes harder requiring a bigger volume.
The density of warm air is less than the colder air above causing it to rise and the colder air to fall.
Simple kinetic theory in a gravitational field gives the basic temperature profile of the troposphere.
Even a single molecule as it rises from the planets surface losing KE and gaining PE tells the basic story.
KE is directly proportional to Temperature and a graph of either against altitude will look the same.
Notice no reference is needed to radiative properties of the gas molecule.
SOD says:
“The first paragraph says (to me) that Motl doesn’t understand the “greenhouse” effect. And the second paragraph on its own might be a good explanation – but in the light of the first paragraph..
First paragraph – “the lapse rate is a pre-requisite for the greenhouse effect”? “Without a lapse rate absorption and emission would be balanced” – true.
But with it, absorption and emission would also be balanced.
My comment – without the lapse rate the surface would be hotter.
And with the lapse rate the surface is cooler.
The back-radiation from the “greenhouse” effect creates the conditions for convection. This is well-explained by DeWitt Payne many times within the comments on this article.
Well, if Lubos Motl wants to explain his point of view, it would be very welcome, and I apologize in advance if I have misunderstood his article.”
Hmmm. I wonder just who is correct about the greenhouse effect here?? Given his background, I really doubt that Lubos does not understand the greenhouse effect. And I really doubt that the backradiation is necessary to cause convection, either. Most of it is probably caused by simple thermalization and lower density of heated air.
I ask again where simple heat capacity “fits in” with the greenhouse gas hypothesis.
And why is the temperature at a given sunny location not higher than the simple solar insolation, by itself, would predict, if we have to add in backradiation? Of course, I can’t put some definite numbers on this, since I still haven’t located the data for hourly backradiation at any specific location; can someone help with that?
Pressure broadening is an amazing thing. Back somewhere on another thread I made some sort of comment about pressure broadening reaching a limit. Not true. I was playing around with MODTRAN to see how low I needed to go on CO2 concentration to get the very low emission that Nasif calculates (~0.1 W/m2). It turns out to be about 5 orders of magnitude smaller or 0.0034 ppmv instead of 380. That produced a very small blip in the graph so I went to Spectracalc ( http://www.spectralcalc.com/calc/spectralcalc.php ) and generated a spectrum at an ambient pressure of 1013 mbar. Then I decided to see how the spectrum changed when varying the pressure over a wide range. So from 0.113 mbar to 101300 in order of magnitude steps, here are the spectra (note the change in the y axis scale from low to high pressure):
101300 would be the same order of magnitude as the surface of Venus.
The lack of cooling on the side of Venus not exposed to the sun is caused by horizontal convection or advection. Winds at the cloud layer have a velocity on the order of 60 times the surface rotation rate so the cloud layer has a rotation period of 4 to 5 days. Then there’s the heat capacity. At the temperature and pressure of the Venusian surface, the heat capacity of the atmosphere would be at least 100 times greater than the heat capacity of the atmosphere at the surface of the Earth. Lots of vibrational modes should be populated at that temperature so there would be an additional degree of freedom for energy storage. At a loss rate of 168 W/m2, it would take a very, very long time for the temperature to drop much.
Altho it remains the case that no-one has presented a quantitative definition of the “greenhouse effect” , I’ve seen that there are a number of people making some insightful points about the conditions necessary for convection , and have even seen the use of the term “graybody temperature” which is so much more appropriate than “blackbody” .
So I’ll pose a thought experiment for those who claim , still with no quantitative equations , that the interior of a sphere , radiantly heated by its surroundings , can become and remain enormously hotter , 16 time the energy density , than those surroundings .
There seems to be no disagreement with the calculation , simply matching the integral of T^4 over the celestial sphere , of the temperature of a gray body in Venus’s orbit of about 390k given a sun of about 5780k subtending about 1/100,000 of the Venusian sky .
Consider instead , a sphere surrounded by a uniform 390k background in all directions . The SB equation comes to exactly the same 390k for the sphere . Does anybody contend that the interior of the sphere can maintain a higher temperature , 2x hotter , than that of its radiant bath ?
That seems to be what is being claimed . Or that somehow , it makes a difference if the sphere is non-uniformly irradiated .
“Does anybody contend that the interior of the sphere can maintain a higher temperature , 2x hotter , than that of its radiant bath ?”
Of course not. That is not what’s being claimed.
Now here is where you’re going to have a problem because from what I’ve seen elsewhere, you don’t seem to believe that a body can have optical properties (reflectivity, absorptivity and transmissivity) that are different at different wavelengths, although such things are common in nature (water is highly transparent in the visible and opaque in the near IR). What’s being claimed is something like that spherical shell contains another sphere and that the outer sphere reflects 76% and is transparent for 24% of radiation with wavelengths less than 4 micrometers and absorbent for wavelengths greater than 4 micrometers. The inner sphere is a blackbody for all wavelengths. Illuminate thr outermost sphere on one side with a solar spectrum with a TSI of 2636 W/m2. So to a good approximation, the inner sphere sees, on average over the whole surface, 0.24*2536/4 = 158 W/m2. If we make the inner sphere superconducting then it will have a constant temperature everywhere. Ignoring the contribution from solar wavelengths greater than 4 micrometers, the system must radiate 158 W/m2 so the temperature of the outer sphere is 229.8 K. The inner sphere, however, sees not only 158 W/m2 from the sun but also 158 W/m2 from the outer sphere so it has a temperature of 273.2 K.
Now we’ll put another shell between the outer shell and the inner sphere and make it transparent at less than 4 micrometers and absorptive at greater than 4 micrometers. The surface of the inner sphere still sees 158 W/m2 and the intermediate shell must radiate at 316 W/m2 so now the sphere must radiate 474 W/m2. Keep adding shells (99 total) and you will eventually get the flux at the surface of the innermost sphere to be 100 times the flux radiated by the outermost sphere.
SOD, a lil further up the thread i asked a similar question to
Leonard Weinstein’s thought experiment… Which you and Nick Stokes have answered… But just due to the greenhouse effect, surly heating a higher layer in the atmosphere would have a similar effect to solar heating at the surface over time… Because, wouldnt you end up with an accumulation of energy from the back radiation in the layers below the heated level, and over time end up from the higher thermal capacity of the lower denser atmosphere causing a similar temperature gradient to what we see, purely from back radiation ?
Maybe irrelevant, maybe not in the case of venus.
“wouldnt you end up with an accumulation of energy from the back radiation in the layers below the heated level, and over time end up from the higher thermal capacity of the lower denser atmosphere causing a similar temperature gradient to what we see, purely from back radiation ?
No.
See Bob Armstrong’s comment and my reply. Absent an internal source of energy, anything inside the altitude where all solar radiation is absorbed will be at constant temperature.
That’s how you make a good approximation of a blackbody. You have an enclosure with the walls at constant temperature and put in a very small hole. The radiation emitted from the hole will have a spectrum very close to that for a blackbody at the wall temperature. The walls of the enclosure don’t even have to be all that absorptive, although it helps to take care of any light that leaks in through the hole. The emissivity will not be exactly 1, but it will be close to 1-hole area/wall area.
Thank you for the response… i can see a few of bobs views vaguely resembling what i was querying about… but i was left not entirly sure which comment in particular was relevant…
“Absent an internal source of energy, anything inside the altitude where all solar radiation is absorbed will be at constant temperature.”
Now, wouldnt the back radiation from a heated layer essentially be the same thing as an internal energy source? Ok so an upper layer is heated to say 280k, and all layers below, over time end up a uniform 280k…. So what youre saying is at that time, emission will equal absorption across all layers? and thus a uniform temperature below the heated layer?
Chris Colose has a post on this topic at Climate Change:
Very interesting; I’ve read this thread, Chris Colose’s, Lubos and Goddard’s plus a few dozen other sources; generally the atmosphere is taken as given with thought experiments about changes in the composition; however no attention is given to how the Venusian atmosphere got in place in the first place; this is not an atmosphere which has ‘gently’ built up due to greenhouse accretion is it? Are the supporters of the Venusian temperature being due to its ghg composition also saying that greenhouse processes could establish the Venusian atmosphere in the first instance?
That’s the runaway part. You start with a planet with liquid water that’s a little too close to the sun. Or maybe it’s ok if the faint sun hypothesis was correct but then TSI increased. Anyway, for whatever reason, the temperature starts going up, more water vapor, higher temperature until eventually the oceans boil away and there is no more liquid water. Now the atmosphere is mostly water. Photolytic decomposition of water to hydrogen and oxygen proceeds and because of the higher temperature and lower gravity, the hydrogen bleeds away to space because the upper tail of the Boltzmann distribution exceeds the escape velocity. Once the oxygen concentration gets high enough, any organic carbon is converted to CO2. With no water condensing, there’s no weathering mechanism to return CO2 to the crust so any volcanic emission stays in the atmosphere. Eventually the surface gets hot enough to cook CO2 out of carbonate rocks near the surface and the entire planetary inventory of carbon exists as CO2 in the atmosphere.
Current TSI at the Earth’s orbit isn’t high enough to cause a runaway even at several thousand ppmv CO2 or it would have by now, like during the PETM for example. How much higher it would need to be is controversial. IIRC, some think as little as 5%. But that’s many million years away if the sun stays on the main sequence.
Cohenite
Many people have written about the evolution of the Venutian atmosphere… Just use google scholar.
While the TSI is not sufficient to cause the runaway you describe, what about an Earth following the impact of the proto-planet that is supposed to have created the Moon? After the impact, the Earth’s surface rocks would be molten and if water was present in quantities similar to today, the atmospheric pressure would have been about 320 bars at the surface.
This steam atmosphere should have been relatively stable as the surface rocks cooled. The top of the troposphere would be at a height of at least 150 km where the temperature would be ~255 Kelvin owing to the formation of water clouds.
The adiabatic lapse rate for steam is about 5 Kelvin/km so the surface temperature should be around 1,000 Kelvin.
My question, how did the atmosphere manage to cool to the point that water could condense at the Earth’s surface. Or did our water get here after the Moon was formed?
There appears to be a multitude of hypotheses on the cause/effects of the greenhouse effect, few of which agree with others. This rings an alarm. The physics of the greenhouse effect desperately needs some empirical evidence/support. Where is it?
DeWitt Payne:
That’s a really interesting graph. And I didn’t realize that spectralcalc would let you change pressure to see the results in the absorption spectrum – which page in spectralcalc are you going to?
http://www.spectralcalc.com/spectral_browser/db_intensity.php is what I was using but it doesn’t have a pressure option.
Sorry, now I’ve realized it isn’t just the home page I have actually clicked your link. I hadn’t seen what this page offered before – I will check it out.
It’s the Gas-Cell simulator module, the link all the way on the left. Select the spectral range (you’re limited to 100 cm-1 for free) on the Observer page then the gas, path length (cm), temperature, pressure and VMR (volumetric mixing ratio, for some reason it took me a long time to figure out what VMR stood for) on the gas cells page and calculate away. It nags you about subscribing every so often but you can ignore it unless you want to do something special. There’s lots of other things you can do if you subscribe. I haven’t been tempted yet.
DeWitt , You’re one of the people I’ve seen interesting comments from . What seems most opaque is the understanding of what to me should be rather simple statements for anyone with even a social science level of math . In the absence of any other quantitative definition of the “greenhouse effect” I have proposed that it be defined essentially as the ratio of the correlations between the spectrum of an object and the spectra of its radiant sources and sinks . How can anybody read that and think I fail to understand the effect of spectra . What drives me nuts is to see lots of word waving about “long” and “short” ends of the spectrum rather than computations with the actual spectra .
Now , in a manner analogous to albedo dropping out of the equation for a uniform gray sphere , the color ( spectrum ) of a sphere falls out for a uniform radiant surrounding .
It seems to me that after saying that no one is claiming that the interior of a a uniformly radiantly heated sphere will come to a higher temperature than that of its surroundings , you proceed to do exactly that with an argument that inner shells reach successively higher temperatures far beyond the exterior temperature despite the common sense fact that all equations of heat flow are from high to low .
Why stop at a hundred layers ? Seems you could keep adding them til the interior melts .
Given what you claim , surely you could construct such an optically layered ball here on earth and have it generate a perpetual current between a thermocouple buried at its center and one at its room temperature exterior .
You can, sort of. But you use a curved mirror or lens to focus solar radiation.
The shell model is a toy. The interior can never exceed the surface temperature of the sun. Without a concentrator, you’d also need a pretty large ball, and a ball isn’t the ideal geometry, to get much power and on a small scale, conductive losses would be a problem. Not to mention that the transmission of solar radiation to the interior and the blocking of IR leaving wouldn’t be perfect so the per layer increase of temperature would be less than the theoretical maximum. Glass absorbs too much near IR while plastic is too transparent in the thermal IR. Besides, the energy out can never exceed the energy in, even if the internal temperature is higher. Any absorption of incoming radiation by the layers reduces the energy out compared to a single layer. I suspect maximum power from a solar water heater is achieved with no more than two layers of glass with the space between the layers evacuated.
It might be an interesting experiment, though, to see if the temperature inside a box increases with multiple layers of glass after correcting for decreased loss of heat by conduction/convection. Maybe one should put the device in a vacuum chamber with a quartz window to solve that problem.
Oh, and I still don’t know what you mean by correlation of spectra. Spectra taken where, surface, space or in between? Looking up or down or both? Total flux or what?
The first to look at is the lumped absorption/emission spectrum of the planet and its atmosphere . The source spectrum is the measured emission spectrum of the sun , and the sink spectrum is the ( insignificant ) 3k cosmic background . A correlation , of course is the dot product of the normed deviations of the spectra from their means ( which have been accounted for by the gray body factor ) . That gives you the actual ratio between the absorptivity from the source and the emissivity towards the sink .
By basic understanding of correlation ( which is the cosine of the angle in vector space between to functions ) , the maximum temperature that an object orbiting the sun can be heated is when the correlation of it’s spectrum with the sun approaches 1 , given that the 3k sink is negligible .
I believe you , yourself , pointed out that if all the radiation from the sun is either absorbed or reflected from a shell , temperature will be uniform within that shell . The only way you get a gradient in temperature is by some portion of the irradiation reaching lower layers and being absorbed there . At every level I would expect a balance in the totality ( sum ) of radiant , thermal , and macroscopic energy density .
De Witt, thanks for the response; a small point about the origin of Venus:
http://www.cosmosmagazine.com/node/1658
O’Neill’s thesis is that surface conditions caused the atmosphere which was then maintained by greenhouse; I hope he is wrong about the first, otherwise it makes SETI a bit of a waste.
Your comparison with PETM set me thinking as well; the PETM was a marvel of weird and sudden temp increase but that temp increase preceded the CO2 increase:
http://www.nature.com/nature/journal/v450/n7173/full/nature06400.html
As well the following tracking up of temp to the Eocene Optimum, where the max temp was almost equal to the PETM, featured CO2 decline.
The PETM is perhaps not a good analogy for Venus.
The other thing about these confounding Venus analogies is this; instead of replacing the atmosphere with other gases, what would happen if the remaining 5% of the Venusian atmosphere became CO2, so that 100% of the atmosphere was CO2; would the temp increase given that there would be in effect no increase in pressure?
This second idea of 100% atmospheric CO2 leads onto the 3rd point; Miskolczi has written his follow up paper, soon to be published; he maintains the OD of Earth has not budged in 60 years; to my layman mental struggles, the OD is a useful measure of the greenhouse effect; if it hasn’t moved then the increase in CO2 has had no effect despite the rain of backradiation. This dovetails with your earlier post on pressure broadening; which is to say, if CO2 has increased but the OD not then why not? The question for Venus with 100% CO2 would be, is there a limit to CO2 heating with a constant pressure?
The most common hypothesis I’ve seen for the PETM are a volcanic event on the sea floor releasing clathrates. I’ve not seen a PETM hypothesis that says that the CO2 level leads temp.
DeWitt Payne, forgive me if this is off the point. I think that increasing optical depth will not increase collision broadening, as this depends on pressure (essentially unchanged if we are thinking a doubling of CO2 in Earth’s atmosphere) and temperature (unchanged, initially at least).
DeWitt,
You continue to omit diffusion from higher altitudes to lower (in all cases), and convection, including that from higher to lower levels due to lateral temperature gradients as increasing the lower temperature. These would eventually heat up the lower atmosphere and reestablish the lapse rate.
Bob Armstrong,
The thing you left out was that if a gas has a particular temperature at a high altitude, and then drops to the surface, it gains kinetic energy from the gravitational potential energy it had at altitude. The lapse rate in fact is just equal to the amount that would be obtained assuming that the absorbed energy occurs at the location where it is emitted to space (it is not necessary for the absorption to actually occur there). The only variations of the lapse rate would be due to phase changes (evaporation and condensation), or the fact that not all of the energy is lost at that high altitude. The only effect of a greenhouse gas and or clouds is to move the location of where the upper atmosphere radiation to space occurs, and thus add low level temperature due to this potential to kinetic energy gain.
What interests me Nathan, is not so much the cause of the PETM but what ended it in such a brief, geologically speaking, period of about 10,000 years. If indeed CO2/ghgs did cause the PETM, and CO2 by itself did not because it did not rise before, then the PETM seems to suggest when CO2 does peak temp becomes independent of it [assuming any dependence] and the climate sensitivity must be less than IPCC assumptions. This is demonstrated by temperature continuing to rise after the post-PETM fall despite CO2 falling.
This relation [sic] between CO2 and temp is what the Beenstock paper promotes; that is, at best it is the first difference in CO2, or its change, not absolute levels, which has any correlation with temp. If that is true then the high levels of CO2 on Venus could not maintain the Venusian temperature.
Re: Weinstein 6/19/2010 2:22pm
Leonard: Thanks for the corrections and excellent comments. I estimated the lapse rate from the slope of the graph instead of calculating it properly. And the simplest scenario would have been to replace the CO2 with a hypothetical non-absorbing gas with the same molecular weight AND heat capacity. Argon doesn’t have the same heat capacity and nitrogen doesn’t have the same MW.
There is about 10^6 times more carbon dioxide in Venus’s atmosphere than Earth’s. Goddard asked us to consider a 90% reduction and, thinking linearly, portrays this as a major change. However, absorption usually varies with the log of concentration, so Goddard’s 90% reduction is only one sixth of the log difference between Earth and Venus. There is a 440 degK difference between the surface temperature of the two planets. If the optical properties of carbon dioxide didn’t change dramatically with pressure and temperature, one might be able to estimate that each factor of ten reduction in carbon dioxide will change the surface temperature about 73 degK. Is there a better estimate? (This seems more reasonable than Goddard’s.)
Despite my frustration with SOD’s overemphasis on radiative transfer, I do not agree with Motl concluding statement: “the “extra hundreds of degrees of Venusian heat” are mostly due to the greenhouse effect is simply wrong”. If the atmosphere didn’t contain a large amount of carbon dioxide under high pressure, radiation at 16,100 W/m^2 would quickly cool the surface of Venus. The high concentration of greenhouse gas means that each upward photon travels only a short distance before it is absorbed. The temperature of the gas that absorbs an upward photon is nearly the same as the temperature of the gas that emits it, making net vertical transfer of energy by radiation inefficient. So convection dominates. The same phenomena applies to the earth’s troposphere at many wavelengths, so that convection dominates there too.
Let’s think about the thermodynamics of the inside of an opaque shell maintained at a constant temperature and no heat or energy source inside the shell. For anything inside the shell to get or stay warmer than the temperature of the shell, heat would have to flow from cold to warm or not flow from warm to cold. I believe that would be a violation of the Second Law. A heat pump can move heat from cold to hot, but it has to have an energy source to do the work. By definition, there is no energy source inside the shell so no heat pump and anything inside the shell that started out warmer than the shell will lose heat until it’s at the same temperature as the shell.
Look at it another way. Is the interior of the Earth at a temperature of ~6,000 K because the surface temperature is ~300 K or the other way around? Is the temperature of the core of the sun millions of degrees because the primary radiating surface is 6,000 K? Lord Kelvin showed in a paper published in 1862 that the sun would radiate all its heat away in less than 100,000,000 years assuming a fixed initial heat content.
http://zapatopi.net/kelvin/papers/on_the_age_of_the_suns_heat.html
Heat will flow down the temperature gradient to the shell until the temperature gradient is zero.
Nick Stokes,
Thermal conduction in gases is actually due to diffusion of molecules. The hotter one have higher kinetic energy. Diffusion from a colder layer at a given altitude down (and diffusion is omnidirectional) would also would cause the colder molecule (lower kinetic energy) to heat up as the potential energy from the greater height in the gravity is converted to higher kinetic energy as it goes lower. This means that gas molecules heat up as they go lower, and this is not from getting heat energy from surrounding gas. The reverse is true as gas molecules diffuse upwards. The result is that heat is NOT transferred from hotter lower layers to colder higher layers in the absence of a driving hot lower layer. The effect of equilibrium is not to make the gas isothermal, but adiabatic, and as pressure drops with altitude (pressure is nothing but the weight of the gas above a give height), the temperature will also drop.
I partly agree with that. But you need to look at the energy flows.
A temperature gradient induces a flux (Fourier’s Law), from conduction and also, more importantly, from gas-gas radiative transfer (eg Rosseland transport). That up flux has to be balanced, in equilibrium.
On Earth and Venus, there are two balancing fluxes. One is SW downwards, which does not interact with the temperature gradient. The other is what I have been calling the adiabatic heat pump, which I think you are referring to here. The motion of gas tends to pump heat downwards when the lapse rate is less than the adiabat.
Your shell cuts off the first down flux. The adiabatic heat pump needs energy, which normally comes from the atmospheric winds, which are in turn driven from heat differentials – conveying heat from a hot source to a cold sink. That can’t be the vertical gradient, which is what the pump actually creates – no machine can run from its own output. So it would have to be from equator-to-pole inhomogeneity or day-night.
But Venus at present does not have much inhomogeneity of that kind. The location of your shell is at almost the same temp, equator-to-pole, noon-to-midnight.
I’ll modify my earlier proposal that the air under the shell would be still and isothermal. There would be some movement and lapse rate. But it would have to be much less. The air would slow until there was enough temp differential around the shell to drive a much reduced circulation, which would yield a very much reduced lapse rate.
To summarise, at present, there is an upfulx in the Venus atmosphere caused by the lapse rate gradient, and this is balanced by the sum of the SW downflux and the heat pump caused by motion. You’ve removed the SW and a source of motion. Only a much reduced motion and lapse rate would remain.
Frank,
I agree with what you say. I think Motl was trying to say that much less greenhouse gas would have only a modest effect, but I think he still implied you need a fairly large amount. The end point is that the greenhouse gas raises the altitude of radiation to space, and the convective driven lapse rate does almost all of the rest. Changing the amount of greenhouse gas just a factor of 2 for Venus would have very little effect. By 10 times, I am not as clear, but 74 K is a reasonable value, as long as the gas mass and specific heats are the same. Venus’s clouds confuse the exact numbers, but not the general story. My smaller number was based on replacing CO2 with Argon, which would result in a larger lapse rate due to the smaller Cp. The combined lower altitude from lower CO2 and lower Cp from Argon nearly balance out.
DeWitt,
I though the core of the Earth is hot due to a combination of slow loss of the initial heat from formation (the lower the delta T the lower the conducted energy, so it is not like the super hot Sun), combined with radioactive decay, and a small amount of tidal effect from the Moon. The large thickness of poor conducting mantel and modest temperature gradient only contribute a very small amount of Earth’s surface energy balance.
DeWitt,
Gravity decreases as you go to deeper levels of Earth, so no potential to kinetic gain is made at depth. The pressure does not cause the high deep Earth temperature. The surface temperature is almost totally due to the value from Solar input with air and water buffers smoothing it out, and modestly increased by the modest greenhouse effect from water vapor and to a much smaller degree, CO2 and methane.
“Gravity decreases as you go to deeper levels of Earth, so no potential to kinetic gain is made at depth.”
That can’t be right? Even though the force of gravity becomes smaller towards the center of the earth (and reaches zero in the end), does surely not mean that there’s no potential energy difference with depth below the surface.
Leonard,
Place a thin spherical shell in orbit with vacuum inside. Just to make things difficult, let’s phase lock the rotation rate to the orbital period so one side always faces the sun. What’s the temperature of the shell if the outside is black for all wavelengths? I contend that the sphere will be isothermal because thermal conductivity inside the shell will be very, very fast. The temperature will be the fourth root of (TSI/0.0000000567/4). If the shell has any reflectivity (r) in the solar spectrum then multiply by the fourth root of (1-r)
Putting an atmosphere and a planet inside the shell doesn’t change the general principle (Second Law) that no part of the interior can be hotter than the highest temperature of the shell at steady state, which would be less than or equal to the fourth root of (TSI/0.0000000567). A reflectivity correction applies here too.
Diffusion doesn’t care much about gravity until the pressure gets very low. Otherwise the atmosphere would stratify into layers by molecular weight, which does happen at very high altitude, greater than about 80 km, when the mean free path of a molecule is measured in kilometers. The 1976 standard atmosphere has the pressure at 70 km as 0.058 mbar so 99.99994% of the atmosphere is below 70 km and stable, non-condensible gases below that level are well-mixed.
Even colloidal suspensions, where the particle sizes and masses are orders of magnitude larger than the molecules of the solvent, diffuse in all directions as long as local g isn’t too high and the particles aren’t too large. You can break colloidal suspensions in an ultra-centrifuge at 100,000 g.
Look at it in terms of entropy. An adiabatic lapse rate is isentropic. In an isothermal atmosphere, entropy increases with altitude. Which way do you think the system wants to go?
“An adiabatic lapse rate is isentropic.”
Not so. It is with respect to convection. But for conduction and radiative transfer, there is irreversible heat transport down the gradient.
DeWitt,
Look at a thin shell (perfect black body at all wavelengths) facing the Sun. It reaches 471 degrees K. It then radiates from both sides at 2,636 W/msquare. Say a single gas molecule hits the surface and develops a velocity relative to the shell to becomes in thermal equilibrium at that level. The gas is so rarified that the molecules can go all the way to the ground about 90 km below with out colliding with another molecule (i.e, the MFP> 90 km). The ground temperature would see the added molecular temperature due to the added velocity from converting the potential energy at the shell to kinetic energy at the ground.
DeWitt,
Ignore the previous. It absorbs 2,636 W/msquare, but initially reaches a temperature of only 389 degrees K and radiates 1,318 W/msquare each way. Upon impact, the gas molecule would obtain a temperature (thermal velocity) relative to the surface of the shell at 389 K. If it then moved to the ground below, it would heat from the conversion of gravitational potential energy, and when it hit the ground, would have an effective thermal velocity with respect to the ground much higher than from 389 K. The law that says one surface can’t be heated more than the source does not apply if other sources of energy (potential to kinetic) are available.
I’ll post my answer in the part 2 thread.
It is amazing that so many people entertain hypotheses that just don’t “pencil out.” If you have 800 wm-2 of direct solar insolation at noon on a nice summer day in Atlanta, and yet you only have the average K&T value of 325 wm-2 of backradiation, the calculated temperature is WAY more than the actual. Even convection can’t explain this discrepancy. WTF?
JAE,
Try your calc with a 24-hour average of solar. The ground and atmos have some heat capacity to consider. Convection takes care of the rest. Or, perhaps all the thermometers and cavity calibrated radiometers are all wrong, although granted, the global average value of back radiation is one of the more difficult components to get right with the key being the global annual averaging part.
George
JAE,
Sorry, but just read some of your earlier posts after posting my previous, my bad. However, you also need to consider the surface-leaving radiation that helps keep the surface air temperature down. In your Atlanta noon example that might about a negative 150 Wm-2 of solar and maybe -400 to -600 W m-2 of IR depending on the surface temperature of course. It is the net surface radiation that you need.
George
Leonard Weinstein:
There is a new post about this thought experiment in Venusian Mysteries – Part Two
George:
“Or, perhaps all the thermometers and cavity calibrated radiometers are all wrong, although granted, the global average value of back radiation is one of the more difficult components to get right with the key being the global annual averaging part.”
Do you know where I can find HOURLY data for the radiometers?
Try this:
http://www-cave.larc.nasa.gov/cave/pages/sam.html
I believe they have 1/2 hour averages for about 60 sites.
G
Uh, thanks, Geo, but I can’t figure out how to get real data from that site. Any hints? I’ve been waiting for over 3 years for this data!!! All I get is this kind of cryptic link.
Tell me, now, just how is it FRIGGING POSSIBLE that such IMPORTANT DATA on backradiation is not readily accessable??? Just where oh where is all this backradiation data that the warmers keep talking about? Where is all this radiometer data showing surface IR from the sky. In short, WTF?? The elegant theorists need to put their money where their mouth is, and show the world some real empirical evidence for the greenhouse effect. The only evidence I’ve seen so far is from Miskolczi. And it shows that additional CO2 doesn’t make a difference.
No data, no dice.
JAE:
-When you have problems understanding a theory you get angry with the people who are trying to explain it.
-When you can’t find data that you want to be available you get angry with people telling you where the data is or what limited data is available.
I said before – there are plenty of blogs to go and be angry on, but this isn’t one of them.
For some reason you have become convinced that because you can’t find back-radiation data from Atlanta and Phoenix in July that this means no one understands the greenhouse effect.
Or because you can’t find radiation data from every point around the world at any specific time of day in a format that works for you in a user-friendly website, that the whole of atmospheric physics has come crashing down.
You have already stated your case that because the information has not been provided to you that this means that the “greenhouse” effect is not real. You don’t need to repeat it – the many visitors to the site have already read your comments and can draw their own conclusions.
If you have a new comment, or would like some information, please make an effort not to be rude. It discourages the many visitors who like to help from helping other people who would benefit.
You have already exceeded your quota.
LOL. What a great way to get rid of dissenting views. Does the word “frigging” really offend you? LOL!
As a relatively new SOD reader, I can *assure* you, it’s not the use of the word “frigging” that makes you seem rude. It goes WAY beyond that. anger is not a valid rhetorical tool, on a site like this, where the vast majority of folks, regardless of what “side” they’re on, are just trying to arrive at common ground.
Said another way: just because *you* don’t understand the science, and/or having a difficult time finding the information, that in NO WAY means the science is wrong. It simply means you have more learning to do.
[…] 22, 2010 by scienceofdoom A long and interesting discussion followed Venusian Mysteries. One commenter posed an excellent thought experiment: Consider Venus with its existing atmosphere, […]
Jonas B1
“Gravity decreases as you go to deeper levels of Earth, so no potential to kinetic gain is made at depth.”
That can’t be right?
+++
It isn’t unless you have an increasing density gradient as you go in.
At the depths you can go in the crust, this is not the case (or, at best, it’s the case in some places and not in others).
Because as you descent, the mass further away from the centre of gravity of the system is self-cancelling: the mass behind you pulling you back is closer, but there’s less of it than the mass pulling you onward, which is further away.
“Tell me, now, just how is it POSSIBLE that such IMPORTANT DATA on backradiation is not readily accessable???”
It is if you know where to look.
But you need to be active in the field specifically to that effect and specifically to that region and the chances of getting someone who is in that very specific field answering you on this blog in the goldfish-level attention span you have is astronomically unlikely.
And how does that follow that just because YOU want it, it’s “such IMPORTANT DATA”? Additionally, why does it prove that the science underpinning the greenhouse effect wrong?
I can’t find the output of mercury traces in the Thailand Nike operations, but this doesn’t mean that Nike don’t produce trainers…
Does anyone have an online reference for the absorption figures for H2O and CO2?
I apologise for a long absence, and also because I have not read all the thread comments. My remarks apply to an equilibrium average planet.
For each chunk of atmosphere, the important radiation number is not the absolute value of absorbed or radiated energy, but the NET. That is what heats the atmosphere radiatively.
For most of the atmosphere this is a very small number.
However for the lowest stratum near the surface, the NET radiation = Radiation_from_Surface ( approximately Stephan Boltzmann) – Back_Radiation_from_Atmosphere – Surface_Radiation_not_absorbed_by_atmosphere. According to Kiehl&Trenberth (see for example, IPCC WG1 Chap1 Fig 1) this is on average around 25W/m^2.
That is, the heating effect on the atmosphere due to absorption of surface radiation is about 25W/m^2.
The effect of the Greenhouse on the Surface is to vary the Back Radiation (this variation is referred to as “forcing”). If CO2 increases, the Back Radiation increases. As the Surface temperature increases, the Surface energy lost as evaporated water also increases.
The result of this is that as CO2 increases the NET Surface radiation absorbed by the atmosphere drops. The NET energy leaving the surface is always constant (and equal to the absorbed solar radiation), but the balance between radiation, conduction and evaporation changes. NET energy transported by evaporation goes up, and by radiation droops by the same amount.
Some people have commented that this explanation is a mathematical trick – that Radiation is overwhelmingly the major transport mechanism, based on the absolute values. But I counter that assertion by asking them to consider a waterless world, with a 1mm vaccuum between the surface and the atmosphere. In that case evaporation and conduction are zero, and it is easy to see that the atmosphere is only heated by the NET absorbed radiation.
At present, according to Kiehl&Trenberth, Evaporation accounts for about 60%, Radiation about 20%, and Conduction about 20% of the Surface energy transferred into the atmosphere.
Virtually all the Surface-emitted photons at the CO2 absorption frequencies are absorbed in the first few hundred metres of atmosphere. I don’t have any numbers for H2O, but I think the absorption is probably a little higher in the atmosphere. (If anyone can direct me to an online table I would be grateful.) As the absorbing CO2 is nearly at Surface temperature, there will be very little NET absorption by CO2. The higher water vapour absorption, being at a lower temperature and accross much wider tracts of frequency, will account for most of the 25W/m^2.
I don’t have numbers for the relative proportions, perhaps someone can do the sums?
[…] 16, 2010 by scienceofdoom During a discussion about Venus (Venusian Mysteries), Leonard Weinstein suggested a thought experiment that prompted a 2nd article of mine. […]
Sorry for you, but your article is disregarding some very important fatcs which totally disprove – as here on Earth – any hypothetical “greenhouse effect” as explaination of the high temperatures on Venus surface.
First of all, you forget to mention that on top of Venus’ atmosphere temperatures are very low, about – 33° C, while on the middle of the atmosphere temperatures are about + 20°C, and so it is impossibile that on Venus there could be a “backradiation” from atmospheric colder gases and CO2 which could “heat” the surface up to 464° C
It is correct – as Steven Goddard said – to consider the very high pressure at the surface, i.e 92 bars compared with only 1 bar on Earth surface as the main factor that can explain the huge temperature.
Morover, Venus density of atmosphere at surface is about 65 Kg/m^3, namely more than 50 times than the one of Earth!
http://nssdc.gsfc.nasa.gov/planetary/factsheet/venusfact.html
But apart from this, in your articles you completely disregard the fact that Venus HAS NO MAGNETIC FIELD, while Earth planet rotates very fastly, and gets a strong magnetic field which keeps away solar winds from heating too much Earth surface, while Venus, having no magnetic field, receives a flow of heating solar winds and plasma heated particles on the surface, and that’s (along with high pressures, and higher solar irradiance) the reason for so high temperatures.
Click to access Temperature_of_Venus.pdf
http://www.astronomynotes.com/solarsys/s7.htm
Therefore, it doesn’t make any sense to compare Venus to Earth on this respect, given the big difference between the 2 planets, and above all, it is completely wrong to regard the 97% of CO2 of Venus atmosphere as the reason for an hypothetical “greenhouse effect” and heating of Venus surface, as the widespread popular (and wrong!) belief maintains.
[…] expansion” and is affected by the amount of water vapor in the atmosphere. See Venusian Mysteries and the following articles for […]
[…] by “adiabatic expansion” and is affected by the amount of water vapor in the atmosphere. See Venusian Mysteries and the following articles for […]
This is just is mind-blowing to me. How can one, just by the mental exercise of arbitrarily subdividing a thick atmosphere into sub-layers, increase the surface temperature to near infinity? (or whatever the (N+1)^1/4 factor may be). The simple formula of surface temperature is based on knowing the temperature of the top layer Te (by equating to incoming solar radiation). If we assume that the atmosphere is a single layer with uniform temperature and opaque to IR, then the temperature at the surface is 2^1/4*Te. If we assume that the temperature is not uniform but gradually increasing towards the surface, that will define the number of ‘layers’. It all appears that another variable is needed to define the temperature profile. I read that the lapse rate in the atmosphere is due to hydrostatic equilibrium, and independent of the greenhouse gas composition. Then the simple model, having the temperature at the top of the atmosphere fixed, and a constant lapse rate down to the surface would also fix the surface temperature as a constant, independent of green house gases. Mind blowing and confusing. I really need to think about this some more and read about it. However the constant temp would be predicated on a constant thickness of the atmosphere, but if the atmosphere was thicker (higher tropopause) then the surface temp would be higher, for a given top layer temperature and constant lapse rate. And what defines the thickness of the tropopause? In a way I am glad I am so confused, it will force me to read more about this.
Like Kung-fu panda says… I am starting at level zero.
A. Vanags:
I thought I had covered your first question by the section under the subheading in the article: So the Surface Temperature is Infinite, you Dummy!!
As the article says..
[Emphasis added].
The idea behind this simple model is to teach. To help the student create a conceptual model of how it might be possible for the surface temperature to get so high.
This is not the actual calculation used to determine what the surface of Venus is expected to be.
For that we need to use the radiative transfer equations. The figure 2 (which is actually the 3rd graphic, sorry about that) from Bullock and Grinspoon shows the results of the calculation. You can see the actual equations which are needed in Atmospheric Radiation and the “Greenhouse” Effect- Part Six – The Equations but they are quite daunting for newcomers. An introduction is given in Part One of that series, which might be a good starting point.
The Feb 7, 2011 post boils down to this:
I actually like that as it makes perfect sense.
However if you read raypierre’s book (pp 299-300 if I remember correctly), he’s adamant that in the troposphere, radiative processes can be disregarded, in first approximation.
So we end up having:
(a) the temperature (and height?) of the tropopause being defined by the surface radiation and the optical thicknesses and transmittance of the atmosphere below
(b) the temperature at the surface being defined by the temperature and height of the tropopause and the adiabatic lapse rate
Hopefully the two approaches mathemagically bring to the same conclusion?
I am trying to resolve in my mind the question of multiple layers in an idealized atmosphere in a ‘thought experiment’. Most explanations I have come across say that given an atmosphere if we subdivide it into n layers, the temperature of the ground would be (1+n)^1/4 that of the effective temperature. Then a typical college exam question would be to calculate the ‘number of layers’ to account for the ground temperature in Venus.
I think the correct statement is that when a layer is ‘added’. So to go from one layer to two is doubling the thickness of the atmosphere and an infinitely thick atmosphere would have infinite ground temperature. But if the thickness is held constant and we subdivide into layers I think would give a different answer. (Since in the first approach the total absorbance increases while in the second it remains constant)
The way I approached it is to assume emissivity=absorbance to be proportional to layer thickness, that the total emissivity Et could be measured, and the total atmospheric height is known. Subdividing Lh into n equal heights the emissivity of each layer is Et/n. Note that here emissivity is only proportional to length, so the implicit assumption is that density is constant. Then after I worked out the equation I would find its limiting value as n goes to infinity and Eo goes to 1.
Working out the equations by hand and verifying numerically and symbolically in Mathcad (I did it up to 4 layers, the formula matches all) I come up with some really puzzling results.
The ratio of Ground temperature to the effective emission temperature is
Tg/Te = ( ( 2 + (n-1)*Eo/n) / (2-Eo/n) )^1/4
Note that for a single layer (homogeneous temperature) the answer is (2/(2-Eo))^1/4. So for a Et =1 the ratio is 2^1/4 and for Et=0 the ratio is 1. However as n is increased to infinity the limiting value is (1+Et/2)^1/4 or (1.5)^1/4 for Et=1.
I think of the number of layers as a ‘mixing ratio’: less layers means a more homogeneous atmosphere, more layers means more distinct temperature differences or gradient.
What puzzles me is that I have gone from eliminating the ground temperature from going to infinity as the atmosphere is subdivided into more and more layers, to now limiting to a value Less than that for a single layer (1.5)^1/4 vs. (2)^1/4
I was expecting it to be higher but limited. So this does not agree with the very high ratio of ground to emitted temperature in Venus.
Note that this also cuts back the maximum temp ratio for the earth. Assuming Te=255 * (1.5)^1/4 = 282 C (instead of 303C) for a non-mixed, layered atmosphere with an emissivity of 1.0 and constant density, this is also troubling.
It would be interesting if the huge difference between the formula ratio of (1.5)^1/4 and the actual ratio in Venus had something to do with varying density.
Another possibility is that is has something to do with absorbing solar radiation (so far this simple model assumes the atmosphere is perfectly transparent to it)
More than likely it is possible I have made a mistake, so I was toying with the idea of using the rte_3_0 matlab program to see if I can reproduce these results and formula, by setting the atmospheric density to be constant. Its going to take me a while to do it and understand the program.. I am not exactly sure if it assumes that each layer is at a uniform temperature since it has high resolution layers and then a coarse set of 30 layers. I would probably have to make them all a single resolution.
Some details worked out for three layers follow:
n=3. Radiation from ground is G, for each layer above it is Ha, Hb, Hc
The radiation balance when looking at the top of the atmosphere is So*(1-A)/4 = (1-Et/n)^3*G + (1-Et/n)^2*Ha + (1-Et/n)*Hb + Hc
The radiation balance at the ground is So*(1-A)/4 + Ha + (1-Et/n)*Hb + (1-Et/n)^2*Hc = G
For layer a: Et/n*G + Et/n*Hb + Et/n*(1-Et/n)*Hc = 2Ha
For layer b: Et/n*(1-Et/n)*G + Et/n*Ha + Et/n*Hc = 2Hb
The resulting ratio of G/(So*(1-A)/4) = (2 + 2/3*Et)/(2 – Et/3)
bolztman*Tg^4 = G
boltzman*Te^4 = So*(1-A)/4
I understand the above is a very coarse model of the atmosphere but I would like to have and understand some simple model that would give the ‘right’ intuitive answers.
I welcome any comments or corrections, I am trying to figure this out.
Frankly , I can’t get past the basic fact that all 3 heat flow modes are from hot to cold . Therefore it is impossible to construct an externally radiantly heated ball such that its interior becomes hotter than that calculated by StefanBoltzmann & Kirchhoff for its surface . For a uniform gray ball in Venus’s orbit , that’s about 328k . I keep waiting for the basic equation which shows how to make heat go uphill . Can you boil your equations down to that nub which makes it clear how Venus’s atmosphere manages that feat so we can build perpetual heat engines based on the principle here on earth ?
NB , I have see a number of claims of substantial geothermal activity on Venus like so many other planets and moons . Given it’s thick atmosphere , that’s the obvious explanation of it having a surface energy density 16 time that supplied by the sun .
I found the flaw in my post above. Please ignore the results and conclusions. I was assuming the emissivity is proportional to path length. This is not right as then it could be greater than one for a very thick atmosphere. I apologize, back to the drawing board, although I still think a closed form limiting solution can be found for infinite number of layers.
I will now try to find a solution for when the transmitibility = exp(-ko*Lt/n), and absorbitivity = 1 – exp(-ko*Lh/n) for each layer. This makes more sense as then absorbitivity goes to 1 as n=1 and Lh goes to infinity.
Update:
I modified the analysis, assuming transmitability = exp(-ko*Lh)
then assuming no reflectivity, absorbitivity = 1 – exp(-ko*Lh)
and emissivity = absorbitivity
Assuming we have a measured total emissivity Et and a height of the atmosphere, the constant ko can be calculated as:
ko = -ln(1-Et)/Lh
When the height is subdivided into n layers, each of height Lh/n, the emissivity of each layer is E = 1 – exp(ln(1-Et)/Lh * Lh/n) which simplifies to E = 1 – (1-Et)^1/n
Then by analyzing the results for 1,2,3,4 etc layers and casting the solution into a single formula, the ratio of Ground radiation to effective radiation is G/(So*(1-A)/4) = ( (n+1) – (n-1)*(1-Et)^1/n )/( (1-Et)^1/n + 1 )
A similar formula can be calculated for the top of the atmosphere. I have not figured out how to compactly show the temperature curve as function of height as n increases.
The limit Ground radiation to effective radiation for a layered atmosphere as n -> infinity is: G/(So*(1-A)/4) = 1 – ln(1-Et)/2
Apparently Et can never be exactly one.. as Et -> 1 the ratio goes to infinity. But given a total emissivity and effective temperature, it provides a Ground temperature.
I havent really searched, so I dont have good numbers for Et for Venus or the earth, but we have surface to effective temp ratios. Plugging them in to see if the above makes sense gives:
Et = 1 – exp(2*(1-ratio))
but ratio = (Tg/Te)^4 (Te defined with E=1, earth ground assumed as E=1)
from the graphs for Venus in the SOD post: Tg = 735.1 K Te = 232 K then the total emissivity is basically Et = 1. For earth Tg=288 Te=255 and Et=0.715
Its dissapointing to find that the equation is so nonlinear near Et=1 that no useful info can be had for the Venus case. I need to research to see if the value of Et=0.715 is even in the ball park for the earth. I will try to see if all of the above can be verified with a program. Now, if there was a simple formula for total emissivity versus H2O or CO2 content, one could have a simple formula for the temperature increase versus their concentration.
I dont know, most probably someone has already done that.. but working this out is helping me (I think) gain an understanding.
Note that the above corresponds to temperatures due to radiative balance and do not include any information about density, gravity, lapse rate etc. At least the ground temp does not goes to infinity as the atmosphere is subdivided into layers. It also does not include solar radiation absorption in the atmosphere which apparently is pretty high for Venus, as only 2.6% of solar energy reaches the surface, nor does include distributed reflection (about 76% currently assumed to be reflected at the very top).
But I think the biggest piece missing is the adiabatic lapse rate effects, now I have formulas for the radiative effect and adiabatic effect individually, I will (when I have more time) try to combine them to see if a closed form solution is even possible for them combined.
If I wanted to show a graph in a post.. how do I do that?
A gray atmosphere may be a reasonable approximation for Venus, but it won’t work for the Earth’s atmosphere.
If you are going to compare Earth and Venus, then you need to compare like to like and look at the temperatures as the same level of air pressure. Fortunately you can go here to see it done in a very concise and coherent way.
Truthseeker:
The author of the article modestly states:
And as the article has so many flaws I am sure it is a parody. I was going to start a parody blog myself but so many people have already done such sterling work that it isn’t necessary.
The author hasn’t realized that all the incident radiation is not absorbed. Competent?
What is the albedo of Venus?
How much radiation per meter squared does Venus absorb compared with the Earth?
If you had actually read this article you would know the answer.
If you pump up a bike tire to a high pressure quickly it gets hot. Does it stay at a high temperature? Why not?
If you pump up a bike tire to a high pressure slowly it doesn’t get hot.
How can this be?
Well, the “self-proclaimed competent physicist”, or clever parody writer, who wrote the article hasn’t realized that the very basic equation pV=nRT means that T can stay the same when pressure is increased because volume can change.
You can see this painfully explained in Convection, Venus, Thought Experiments and Tall Rooms Full of Gas – A Discussion.
My challenge to you Truthseeker is to read this article and then find a thermodynamics textbook which says that high pressure gas is automatically at a higher temperature than a low pressure gas.
What keeps it at a high temperature?
The surface of Venus is radiating at a much much higher rate than the incoming radiation at the top of the atmosphere. How is this possible?
What stops the surface cooling down rapidly? The pressure?
How does high pressure atmosphere stop the surface radiating? Or if it doesn’t stop it radiating, how does it continually add energy?
Concise and coherent?
Clearly a parody blog and you have been taken in.
If that’s a ‘parody blog” (and I’ll wager it’s not), I am–what’s the word?–GOBSMACKED at Huffman’s…cheek? Chutzpah? Smarminess? It is so *mind-bogglingly* full of factual errors, non sequiters, and outright logical fallacies that a Ph.D. program could be designed just refuting it!
SoD : rather than what that guy got wrong it’d be interesting for you to comment why he got the “right” numbers for the limited heights he computed about.
omnologos:
You mean that if I multiplied one parameter by my shoe size and got the right answer to a question I was interested in it would be interesting (to you) to see why?
Let me ask you another question – if I send someone to the casino to play roulette with $1000 and at the end of the night they come out with $1100 does that mean they have a winning system, or they were lucky on the night?
Let me ask you yet another question – if someone ignores the actual reflection of solar radiation and (some other important factors) and as a result “calculates” the actual value of some important parameter, does that mean that reflection of solar radiation no longer exists?
1. Reflection of solar radiation must be taken into consideration in a calculation of planetary energy balance.
2. The temperature of the atmosphere above the surface is not determined by Stefan-Boltzmann’s law. It is determined by:
– the lapse rate
– the height at which we want to know the temperature
– the actual effective average height of emission to space
If a self-declared “competent physicist”, or entertaining parody writer, ignores all of these factors and we ascribe value to “getting the right answer in an amusing way that is nothing to do with physics” then the joke is on us.
Luckily I think that although many people don’t understand item 2, just about everyone can get item 1.
76% of solar radiation is reflected from Venus, so combining that with distance means that Venus absorbs only 2/3 of the solar energy that Earth absorbs, despite being closer to the sun. Observant readers will note that this invalidates the “concise and coherent” analysis in the linked article.
Obviously a competent physicist wouldn’t make this kind of super-basic mistake because that would make them some kind of buffoon, so this clearly marks out the writer as the intelligent producer of a parody blog.
I take my hat off to him.
I keep toying with the idea of producing my own parody blog, but when I see this kind of outstanding work I realize that others are already head and shoulders above anything I could produce!
I have now had a scan through the some of the comments on the parody blog..
And being as we are at the end of a long thread, I hope readers won’t mind my taking up “print space” with a comment from Celeste who says, playing along beautifully with the theme of the parody:
In reply, the author says:
Beautiful, outstanding, breath-taking.
Take a bow. The world needs more parody writers and I just ask, is there anyone better than this?
Sometimes I wish you could fly low…I’m still unsure _why_ he got “lucky on the night”. The fact that he did suggests there might be something interesting in the way he managed to get the “magical” value of 1.176. But maybe not.
The ~ 328k temperature calculated for a gray ( flat spectrum ) body in Venus’s orbit , which can be simply calculated by summing the energy impinging on a point in the orbit , is the “null hypothesis” temperature , deviations from which need to be explained . Either non-flat spectrum , or non uniform “gray value” ( I’ll use that term instead of “albedo” because that term as generally used is confounded with spectrum ) between day side and night side will change that equilibrium temperature .
It’s absolutely true that static pressure does not enter equations for heat flow .
However it is most fundamental that all heat flow equations are from hot to cold . Therefore , there is no mechanism by which the interior of a sphere can have a higher equilibrium temperature than that calculated for its surface .
The only explanation for Venus’s surface temperature being more than twice that of a gray ball in it’s orbit is internal heating held in by a very thick , insulative atmosphere .
[…] ideas are hardly new as PaAnnoyed, Steven Goddard, Harry Dale Huffman, Leonard Weinstein, gallopingcamel and others have made similar assertions. N&K boiled it all down to a few […]
[…] [1] https://scienceofdoom.com/2010/06/12/venusian-mysteries/ http://wattsupwiththat.com/2010/05/06/hyperventilating-on-venus/ […]
Can someone explain this to me?
From the temperature and pressure profiles for the Venusian atmosphere, you can confirm that, at the altitude where the pressure = 1000 millibars, which is the sea level pressure of Earth, the temperature of the Venusian atmosphere is 66ºC = 339K.
This is much warmer than the temperature at the surface of the Earth (at pressure = 1000 millibars), which is about 15ºC = 288K. (-snip-)
Venus is closer to the Sun, and gets proportionally more power from it. Earth is 93 million miles from the Sun, on average, while Venus is only 67.25 million. Since the intensity of the Sun’s radiation decreases with distance from it as 1 over r-squared, Venus receives (93/67.25) squared, or 1.91 times the power per unit area that Earth receives, on average.
Since the radiating temperature of an isolated body in space varies as the fourth-root of the power incident upon it, by the Stefan-Boltzmann law, the radiating temperature of Venus should be the fourth-root of 1.91 (or the square-root of 93/67.25) = 1.176 times that of the Earth. Furthermore, since the atmospheric pressure varies as the temperature, the temperature at any given pressure level in the Venusian atmosphere should be 1.176 times the temperature at that same pressure level in the Earth atmosphere, in the two atmospheres. In particular, the averaged temperature at 1000 millibars on Earth is about 15ºC = 288K, so the corresponding temperature on Venus, should be 1.176 times that, or 339K. But this is just 66ºC, the temperature we actually find there from the temperature and pressure profiles for Venus.
(So, is there no greenhouse effect? Why are the two planets just behaving the same. )
[Note: The derivation of the radiating temperature above is for absolute temperature, in degrees Kelvin (K), so the 1.176 factor relates the Kelvin temperatures, not the Celsius temperatures.]
Ed Burgener,
Did you read the article?
The Earth has an albedo of about 0.3 so absorbs approximately 70% of the incident solar radiation, whereas Venus has an albedo of 0.76 and so absorbs approximately 24% of its incident solar radiation.
1. Earth’s absorbed solar radiation, averaged over the planet’s surface area = 239 W/m²
– and the measurement of emitted radiation from the climate system, globally annually averaged, is about this number.
2. Venus absorbed solar radiation, averaged over the planet’s surface area = 158 W/m²
So right away we see that Venus should be colder than the Earth.
Simple stuff. Please add your comment and we can move forward.
Given the asserted 0.24 absorptivity with respect to the sun’s spectrum , using the same crude assumption that it radiates as a black body at its orbital temperature of about 328k , we get an equilibrium temperature of
0.24 ^ % 4 />/ 0.699
328 * 0.699 />/ 229.6
I’ll soon be releasing a paper building on the most basic material at http://climatewiki.org/wiki/Category:Essential_Physics working thru the computation of the equilibrium spectra for any set of spectra .
Bob Armstrong,
Asserted?? Measured at 0.3. See The Earth’s Energy Budget – Part Four – Albedo.
I have no idea what the rest of your comment is trying to say, but past experience tells me it will be a waste of time finding out.
You just asserted an albedo of .76 in the comment I replied to . I’ve seen other similar numbers , I was doing the calculation for Venus . I simply do the ubiquitous calculation on the assumption used to create the 255k temperature for a naked earth , that at long wavelengths , it emits as a black body . The K notation seems simple enough that I thought it was unambiguous .
In words , 0.24 raised to the reciprocal of 4 give 0.699 . 328k , which is the gray body temperature in Venus’s orbit ( the temperature corresponding to the total energy density at a point in its orbit ( essentially all from the sun ) ) times 0.699 is 229.6
I’ve actually been following some of your posts on atmospheric dynamics and plan to revisit them when I get to such things .
I keep getting puzzled by Dale Huffmans blog. Such as “The venusian atmosphere is opaque to visible light, thus the greenhouse model for earth does not apply. However, the temperature and pressure profiles of Venus and Earth are similar, but of course the venus atmospheric pressure on the ground is approximately two orders higher than on
the Earth. At 50 km altitude the venusian atmospheric pressure corresponds to the normal pressure on the Earth with temperatures at approximately 37 Celsius degrees”
and
“Venus’s atmosphere is really warmed by 1.91 times the power per unit area as is Earth’s atmosphere, despite its much larger albedo, and precisely as expected soley from itrs smaller distance from the sun.”
How can his empircal data co-exist with your theory? He says that the data proves that you are wrong. I am baffled!
your help on this is apppreciated.
The thought exercise on this page is dumb to put it mildly.
Firstly. Except at high altitudes, Venus is mostly opaque to most light at all frequencies.
Secondly, Venus has terrific planetary winds which spiral from the upper atmosphere to the poles.
Thirdly. The premise That the hot gas on the surface is radiation; is complete twaddle. Its a container of energy like a pressurised container that is not gaining nor losing any heat. Or a bolt screwed down over a spring.
It’s given as fact the upper gas layer is heated via the Sun and spirals to the poles (at a great rate) whereupon it spirals down the polar high pressure inversions and heats up. Yes it fights it but is not enough to overcome the equators low pressure and Solar heating. The poles on Venus are every bit as hot as the equator. Only a fool would attempt to explain this away.
The polar gas at ground level makes its way back to the low pressure zone at the equator and climbs back up losing some heat but also gaining more Solar energy to make up for the loss and keeping the Carnot cycle going.
Galloping Camel and the guys who define a body of gas at a specific heat volume being compressed to increase the heat have hit the bullseye.
As a reaction to the above message but not really as an answer to it specifically I copy here a message that I just wrote on another blog.
The discussion of that SoD thread is also good evidence on the difficulty of agreeing what’s the best way of describing the GHE in the Earth atmosphere where several simultaneous effects contribute comparable amounts to the overall effect:
– changes in the radiation from the upper troposphere to space
– changes in the influence of clouds (even without any change in cloudiness) due to the change in transparency of the atmosphere
– changes in the amount of radiation that escapes directly from the surface to the space
The case of the Earth is so complex because the mean free path of IR spans the whole range from very short (meters) to more than the thickness of the atmosphere. The whole range is applicable to a significant enough fraction of radiation to invalidate both the assumption of thin atmosphere and the assumption of very opaque atmosphere as a quantitatively valid way of describing the physics.
For most of Venus atmosphere the assumption of very opaque atmosphere is valid. That means that radiative heat transfer can be considered as a form of conduction of heat. The diffusion equation is valid for it and the conductivity is the less the shorter the mean free path is, i.e. the less the more GHG’s there is. The resulting effective conductivity is low and leads to very high temperature gradients if it’s the strongest heat transfer process. Under such conditions very weak heating power could lead to very high temperatures.
In the high pressure gas of the lower Venus atmosphere lines are very broad and continuum absorption is also important. All the IR radiation from the surface gets absorbed at very low altitude and then transferred further by the conduction-like radiative process and convection. Because the radiative heat transfer is so strongly inhibited, convection dominates when it’s present.
Convection cannot exist without an atmospheric heat engine that drives it. Even weak solar radiation that reaches the surface is enough to drive some convection. (The geothermal heat does the same but is likely to be much weaker than the little solar at Venus surface.) Convection that reaches the Venus surface may be driven also from above by the heat engine that operates between the altitudes where most of the solar energy is absorbed and upper levels which radiate directly to space. There’s probably more scientific knowledge on the importance of these heat engine processes for the convection in lower Venus atmosphere but I don’t know about that.
Whatever the driver of the convection is, the outcome is the same: a strong lapse rate down to the surface and a very hot surface.
===
I add one more recent reference relevant to the above (Tagagi et al. 2010). I learned about this reference after writing my above comment.
Andyj,
The opacity of the Venusian atmosphere to visible light is often overestimated. In fact, it’s about as bright at the surface as the Earth surface on a cloudy day. Peak insolation at the surface is about 40 W/m². That’s easily enough to drive the surface temperature to its present levels given the low total thermal conductivity of the Venusian atmosphere. Convection at the surface is slight according to observations taken by the Venera 9 probe.
http://en.wikipedia.org/wiki/Venera_9
DeWitt ,
Very interesting link to Venera flights . Any link to more quantitative data ?
“Peak insolation at the surface is about 40 W/m². That’s easily enough to drive the surface temperature to its present levels given the low total thermal conductivity of the Venusian atmosphere.”
Is it ? Can you show the calculations ? I still have never seen the crucial equations which show how to make a ball such that its interior maintains a higher energy density than that calculated for the flux at its surface . The energy density for a point in Venus’s orbit corresponds to a temperature of about 390k . The surface is about 758 , about 15 times the energy density .
I’m dying to see the computation ..
Bob,
Only little energy is needed to keep a well insulated body hot. Venusiian atmosphere provides such a good insulation.
Pekka ,
Ok , then show me the computations .
That energy that reaches the surface is just a small portion of that which is not reflected from the planet , And the total impinging on the planet corresponds to just 390k .
I think a much more believable explanation in lieu of a quantitative proof , and given the lava flows seen by Venera 10 , is that like many other planetary bodies , Venus is geothermally active and the very thick atmosphere , as DeWitt says , slows the escape of the heat .
Check the Tagagi et al paper. I gave the link to that at the end of my lengthier message.
The atmosphere provides an equal insulation for heat from the sun to Venus surface as it gives for heat from Venus interior. For the Earth the geothermal heating is about 0.03% of the solar heating. Even if the ratio were much higher for Venus it’s probably still very small.
Thanks Pekka .
I’ve bookmarked the paper to try to extract the essential relationships from the specifics of Venus — when I have time , which is likely not to be soon . This is the absolutely crucial relationship for any claim that surface warming can be essentially unlimited given an atmosphere with the appropriate stack of spectra .
I’m still highly skeptical to say the least that any structure can make heat stay up hill other than the small amount calculated for the gravity well . I fail to see how it can be not possible , even as a thought experiment , to create a heat engine between that extremely hot surface and the surrounding space .
BTW , a surface wind of 3.5m%s , 12.6km%hr isn’t exactly a storm . I think another fact which prima facie argues for internal rather than up hill flow of external heat is that the whole surface is said to be essentially uniform temperature from pole to pole and from dusk to dawn 1400 hours later .
You are right in saying that gravity is essential. It’s essential because it creates the density profile and the pressure gradient of the atmosphere. Without gravity the whole atmosphere would not be there (or even the planet itself).
The paper of Tagagi et al tells essentially what makes the radiative heat transfer weak in the lower Venusian atmosphere. Even in the Earth atmosphere the radiative heat transfer is so weak that the Earth surface would be much (some 45 C) hotter than it is, if the only ways it can loose energy where conduction and radiative heat transfer. In absence of convection and latent heat transfer both the Earth atmosphere and the Venus atmosphere were very good layers of insulation, good enough to maintain a hotter than present surface when the surface is heated by the amount of solar energy it’s being heated presently.
Here enters the convection. Convection is a very efficient mechanism for transferring heat, but only when it’s present. Convection can persist only when there is a large enough lapse rate. The lapse rate must be locally at least the adiabatic lapse rate that applies to the composition of atmosphere at that point. If the lapse rate is smaller an parcel of air going up cools to a lower temperature than surrounding air by adiabatic expansion. Similarly a parcel going down heats by adiabatic compression to a higher temperature than surrounding air. Both of these phenomena stop the motion of the parcel. Gravity is an essential factor in the above because gravity is the reason for the pressure gradient.
Convection helps greatly in estimating the surface temperature because calculating the adiabatic lapse rate is easy as soon as the specific heat of the gas is known. Two other peaces of information are needed: the height of the convective atmosphere (the troposphere) and the temperature at the top of the convective layer. This temperature is somewhat less than the effective radiative temperature of the planet. Thus a rough estimate of that is easy. What’s more difficult is estimating the height of the troposphere from theory. It’s determined as the altitude where the temperature gradient without convection is equal to the adiabatic lapse rate. The gradient without convection is high at low altitudes and small very high up. At some point it crosses the adiabatic lapse rate. This is the height of troposphere. That calculation requires the absorption properties of the atmosphere that Tagagi et al have studied.
But here we have the option of using empirical data. We can find out empirically both the height of the troposphere and the temperature at the top of troposphere. Using these empirical values we can at least understand why the surface has the temperature it has as long as the top of troposphere is as it is.
This presentation by Caltech JPL scientist Dave Crisp tells about the present knowledge about Venus GHE
Click to access crisp.pdf
Pekka , Thanks for the meat . It’s all too hard to find in the web discussion drowned out by arrogant ignorance . Your http://pirila.fi/energy/ is pleasantly objective . I’ve been thru Helsinki to an APL conference in Leningrad ( In any case his statue was in front of the train station ) in 1992 . Even crashed there overnight on the way back with a friend I’d met at the conference .
I’ve begun to build a vocabulary in an APL expressing the physics of planetary temperature in succinct executable notation . So far I’ve only gotten as far as How to Calculate the Temperature of a Radiantly Heated Colored Ball : http://cosy.com/Science/ColoredBalls.html . I have not yet added this material to Heartland.org’s http://climatewiki.org/wiki/Category:Essential_Physics which I’ve initiated . If you are interested contributing algorithms of essential computations in some array capable language , let me know .
I’m not sure when I’ll have time , I’m trying to concentrate on my 4th.CoSy language right now , but I will be working on extracting that differential which causes 2 domains at different temperatures to have 0 net energy flow between them .
Bob Armstrong,
I’ve calculated the radiative transfer to and from the surface using the line by line RT program at spectralcalc.com and the high pressure line database there. At the surface temperature of Venus there’s only about a 5 W/m² excess of LW radiation up from the surface compared to LW radiation down from the atmosphere assuming an adiabatic lapse rate. The lack of erosion observed indicates that sensible convective/conductive heat transfer from the surface is low and latent heat transfer is non-existent. So there’s enough solar energy averaged over the planetary surface to maintain that low radiative heat flow through a very high resistance resulting in a very high surface temperature. The spreadsheet I used for calculation is huge because Spectralcalc produces lots of data points. I’m reluctant to go back and try to open it now.
Here is one more recent paper on related calculation
Click to access LeeAndRichardson11.pdf
The radiative heat transfer is the one to be calculated. Conductive heat transfer is always very weak in a gas and the temperature profile near the surface adjusts automatically to support the adiabatic lapse rate. Convection is very efficient when the lapse rate would exceed the adiabatic one in some area, but vertical convection cannot lead to much lesser lapse rate than adiabatic (corresponding to the local composition of gases).
DeWitt , could you point me to that “RT program” . I’m at least bookmarking all these references .
You will see on my http://cosy.com/Science/ColoredBalls.html how it’s reasonable to talk about short wavelength versus long , but why to actually calculate temperatures you need the actual full spectra of what ever you are talking about . Does anyone have a link to the full spectrum of the earth as seen from a distant probe ? How different from that is our averaged surface temperature ?
And does anybody have a full spectrum for Venus ? Not just the lumped albedo with respect to the sun . But as crucially , what is the rest of its spectrum ? What’s the long wave portion ?
One of the first graphs I made when this battle for rationality was heating up back in 2008 is http://cosy.com/Science/PlanetTempPlotT250.gif , which shows that all the other inner planets follow their gray ball temperature rather closely . ( We are just 9 or 10 degrees ( 3% ) warmer than a flat spectrum ball in our orbit . ) My bet is that that the limped earth’s spectrum explains that down to a degree or so .
Using values from NASA’s Venus Fact Page , Venus is | 737 % 327.65 |>| 2.25 times hotter than that of an opaque gray ball in its orbit . That means 25.63 time the energy density at its surface as outside . Given that it’s Bond albedo is 0.9 , thus it’s absorptivity 0.1 , its emissivity in the long wavelength band must be | 0.1 % 25.63 |>| 0.004 .
Is that everbody’s contention ?
A little big of geothermal heating like so many other planets exhibit would sure ease that ratio .
http://www.spectralcalc.com
A subscription is required to do anything interesting. There is a free line-by-line RT program available, but using it isn’t exactly intuitive. Search on LBLRTM. You would also need the high pressure line database. I’m not sure where to get that. HITRAN you can get for free.
[…] trying to prove me wrong with big long equations and complicated, point by point refutations and same old tired theories in a desperate attempts to prove my dead simple explanation wrong. My idea is so radical, even some […]
I only recently became aware of this site, and this informative article is the first one I have read. I can see that there are a number of posts on interesting topics and I look forward to reading them.
In this post, however, it appears to me that the line of reasoning for one of the topics goes off track here:
“But for those who believe that high Venusian atmospheric pressure and the ideal gas laws cause the high 730K surface temperature – they have to explain how the heat is transferred to the surface so that it can radiate at 16,100 W/m².”
That reasoning is not consistent with a key point of the pressure based theories, which is that the resulting surface temperature is established right *at* the surface due to the atmospheric pressure in combination with solar insolation. Therefore there is no need to have a transfer mechanism to move heat *to* the surface. The heat is already there, from the get go.
I do not mean to imply that this shows the pressure based theories to be more correct than the GHE based theories, only that one cannot rule *out* the pressure based theories based upon the line of reasoning presented in the article.
While there are several references I could cite, the one most directly relevant is Nikolov and Zeller (2017):
https://www.omicsonline.org/open-access/new-insights-on-the-physical-nature-of-the-atmospheric-greenhouse-effect-deduced-from-an-empirical-planetary-temperature-model.php?aid=88574
This has the added bonus of having, in effect, a paper-within-the-paper in the form of an empirical study. This study derives an accurate estimation formula for the long term average near surface atmospheric temperature for certain planetary bodies, including Venus, as a function of basic planetary parameters. These parameters do *not* include the greenhouse gas concentrations nor any other parameters closely associated with the greenhouse effect.
No, you’ve missed the point or perhaps I wasn’t clear enough in my comment. In the absence of radiation from the atmosphere to nearly balance the radiation from the surface, the surface would rapidly cool by radiation to space. Pressure heating by gravitational collapse is a one time event, it is not a source of continuing energy input.
By the way, your reference is severely flawed by questionable assumptions. It’s not worth my time to point out all the errors.
DeWitt,
Although you had some of the last comments posted here, I was actually responding only to the article itself, which I had seen just recently.
I could have pointed to a different reference to help readers understand what the pressure theories involve, Holmes (2018):
https://www.researchgate.net/publication/324599511_Thermal_Enhancement_on_Planetary_Bodies_and_the_Relevance_of_the_Molar_Mass_Version_of_the_Ideal_Gas_Law_to_the_Null_Hypothesis_of_Climate_Change
Perhaps we can discuss Nikolov and Zeller another time. People can legitimately debate how they estimated certain planetary parameters when the numbers they needed were not directly available. Otherwise, I have seen several people assert there were flaws in the paper, just as you have done here, but upon digging deeper the assumed flaws turned out to be only a misunderstanding of what the paper actually was stating.
Even if I had never suggested any supplementary clarifying reference for background purposes, leaving the representation of pressure theories to the two references already cited in the article, the point of my comment remains: the rationale presented for dismissing the pressure based theories doesn’t apply to what those theories actually incorporate.
DeWitt,
Regarding: “Pressure heating by gravitational collapse is a one time event, it is not a source of continuing energy input.”
I would agree with that for situations where there is no supplementary energy input. In this case there is solar input operating upon the newly compressed atmosphere, which is now at a higher pressure, and where the force of compression (gravity) is sustained. The higher pressure atmosphere will do a better job of transferring heat from the surface into the atmosphere than its lower pressure predecessor would have.
Looks like my second reply to the same post, because there were two different aspects to it, erased the first reply. For future reference, is that how the site works?
On January 24, 2020 DeWitt Payne also wrote:
(https://scienceofdoom.com/2010/06/12/venusian-mysteries/#comment-149660)
“In the absence of radiation from the atmosphere to nearly balance the radiation from the surface, the surface would rapidly cool by radiation to space.”
OK, no problem. Such downwelling radiation is completely consistent with the pressure based theories of planetary near surface temperatures.
In fact, Nikolov and Zeller would likely agree with your point. As they explain in the introduction to their 2017 paper, one of the motivations for their work was to try to come up with a better explanation than GHE provided for the observed downwelling radiation.
Based on the strong predictive power of the formulation they came up with, it appears to me that they succeeded.
On January 24, 2020 DeWitt Payne wrote, lastly:
(https://scienceofdoom.com/2010/06/12/venusian-mysteries/#comment-149660)
“By the way, your reference is severely flawed by questionable assumptions. It’s not worth my time to point out all the errors.”
When I have come across similar comments in the past, I usually suggest focusing on only one (at a time) of the two very distinct aspects to the paper. Here, I want to focus initially only on the empirical temperature study, the “paper-within-the-paper” as I referred to it in my first post. This is the part describing how they came up with their estimation formula for the long term global average of near surface atmospheric temperature for certain types of planets.
There is room for debate over how well Nikolov and Zeller came up with the planetary parameters they needed in those cases where the official data was either incomplete or inconsistent. It looked to me that they made a legitimate good faith effort to come up with the most accurate values that they could.
Apart from that aspect of their work, I have read a number of attempts to undermine the process by which they arrived at the formula and/or the characteristics of the formula that resulted. None have been successful.
__________
DeWitt,
I am not saying that the concerns you alluded to are not legitimate criticisms. I can only say that if you should decide to post some shortcomings regarding the estimation formula aspects of Nikolov and Zeller’s work, they will be received with great interest on my part, taken seriously, and considered carefully. Then I will either acknowledge my agreement with them or let you know if I have difficulty accepting their validity.
OK, here’s the problem. The Earth is a reasonable approximation of an isothermal sphere as far as emission from the top of the atmosphere. If one integrates actual emission over the globe and converts to an average temperature, you get a brightness temperature only a couple of degrees K lower than if you assume it’s isothermal and emission is constant everywhere.
That is not at all true of the moon. The lunar average surface temperature is quite a bit lower than if you assumed an isothermal surface because the equator is hotter and the poles are much colder, resulting in a much lower calculated average temperature. That’s where the bogus 90 degrees estimate comes from. The reason that the Earth is nearly isothermal has very little to do with the spectral properties of the atmosphere. It’s mostly heat transfer from the equator to the poles by circulating air and water.
Therefore, comparing an Earth with an optically transparent atmosphere to the atmosphere free Moon is completely beyond the pale. It isn’t good science. Self reference, that is accepting the assumption that the GHE is 90K, not 33K relies only on a paper by one of the authors. It is hardly accepted science. The etiquette rules of this site state: “Basic Science is Accepted – This blog accepts the standard field of physics as proven.” While Nikolov and Zeller don’t quite descend to the level of the worst arguments, they’re definitely in the ballpark of Miskolczi ( https://scienceofdoom.com/roadmap/miskolczi/ ) and his ilk.
I haven’t read your other replies and I doubt that I will. It’s never a good sign to have multiple consecutive posts.
You had raised three separate criticisms in your comment replying to my first post. I thought it best for clarity’s sake to address each one of the three in a separate comment. That means there are necessarily several posts in a row in order to do that.
Rotation rate also has an effect. A planet or sphere phase locked to the sun and with the rotation axis perpendicular to the plane of the ecliptic so that one side was never illuminated but with no atmosphere or ocean to transfer energy would have a lower average temperature than a rapidly rotating sphere. Gerlich and Tscheuschner have a section in their paper that deals with that. ( https://scienceofdoom.com/roadmap/gerlich-tscheuschner/ ) Relative to the sun, the Earth’s moon rotates slowly so that the side not facing the sun can reach very low temperatures, lowering the global average temperature.
But none of that has anything to do with the atmospheric greenhouse effect on either the Earth or Venus. Hence, the effect of greenhouse gases in the Earth’s atmosphere is indeed about 33K, not 90K.
RationalClimate,
The publisher of the Nikolov and Zeller article you cited, OMICS Group, has been fined $50 million by the US Federal Trade Commission for deceptive practices.
https://arstechnica.com/science/2019/04/ftc-hits-predatory-scientific-publisher-with-a-50-million-fine/
There are several critiques of that paper online.
http://rabett.blogspot.com/2017/08/making-elephant-dance-as-performed-by.html
DeWitt,
Thanks for your reply and the citations, all of which I have studied.
===========> OMICS
I was unaware of the OMICS situation and appreciate being apprised of it. I respect the implication that any paper published under those auspices needs very careful scrutiny.
Fortunately for my own peace of mind, all of the key results in the N&Z paper are transparently developed within the paper, and the important numerical values can be independently verified against external sources with a scientific calculator or a spreadsheet. No computer models are required. So — I feel satisfied with my understanding of what they did.
===========> 90K vs. 33K
Regarding your previous point about the difference between 90K and 33K, that is an apples-to-oranges comparison. The 90K N&Z figure relates an *airless* planetary temperature to earth’s current average temperature. In contrast, the 33K figure implicitly assumes an atmosphere, but one without GHGs. This is because the earth parameters used for computing the 33K value include its current albedo, which depends on clouds and oceans.
(As an aside, I saw mention on Twitter of a newer paper that calculates a value of 66K+. I want to track that down and study it. If the work is solid, then N&Z may no longer be the only authors citing a value >33K.)
===========> ELI RABETT
The Eli Rabett column you cited raises a number of different issues, and I will address each one in turn.
http://rabett.blogspot.com/2017/08/making-elephant-dance-as-performed-by.html
》OVERFITTING
First, Eli incorrectly states that the N&Z work involved a free choice of fitting form. As N&Z point out: “We specifically avoided non-monotonic functions such as polynomials because of their ability to accurately fit almost any dataset given a sufficiently large number of regression coefficients while at the same time showing poor predictive skills beyond the calibration data range.”
Eli at one point had a related column where he fit a polynomial to some example data points. I had an extended Twitter exchange with him about that and demonstrated that it was mathematically impossible to achieve a good fit to his data by using only monotonically increasing functions.
So his quote from John von Neuman:
With four parameters I can fit an elephant, and with five I can make him wiggle his trunk.
does not apply for the functional forms to which N&Z restricted their analytical work.
》REFERENCE PRESSURE
Eli correctly quotes N&Z stating: “the selection of Pr is a matter of convention”, yet he appears not to fully appreciate that, unlike the concept of a reference temperature, Pr serves a mathematical purpose and does not relate to any particular planet. As a consequence, four paragraphs of discussion in the column about hypothetical planetary variations in Pr are n/a wrt the point being made.
》GHE-DRIVEN TEMPERATURES
The next point cites a paper by Arthur Smith, which overall provides a good analysis.
https://arxiv.org/abs/0802.4324
Eli’s statement is that: “Arthur showed, as was well known, that the average temperature of the surface of a rotating planet without greenhouse gases has to be less than the effective temperature.”
But in fact Arthur *didn’t* show that. His otherwise logical conclusions were based on an incorrect assumption that occurs in the paper right here, in the second sentence of this paragraph:
IV. INFRARED ABSORPTION IN THE ATMOSPHERE (4th paragraph)
“Net energy flux is determined by the radiation that gets into space, not what leaves the surface. The only way for a planet to be radiatively warmer than the incoming sunlight allows is for some of that thermal radiation to be blocked from leaving.”
By using the word “only” Arthur has simply *assumed* *away* the entire Nikolov and Zeller hypothesis about pressure-enhanced warming. In fairness to Arthur, the N&Z work was years away from being published at that time, and the common assumption in climate science back then was that radiative was all that mattered, so he cannot be faulted for making that assumption.
What we know *now* is that the N&Z formulation provides very strong predictive power, normally the gold standard for a good scientific theory, so we should not now be assuming it away without a clear justification for doing so.
N&Z 2017:
https://www.omicsonline.org/open-access/new-insights-on-the-physical-nature-of-the-atmospheric-greenhouse-effect-deduced-from-an-empirical-planetary-temperature-model.php?aid=88574
》MOON SURFACE ASSUMPTION
Lastly, Eli criticizes the use of an assumption in this paper:
On the average temperature of airless spherical bodies and the magnitude of Earth’s atmospheric thermal effect
https://springerplus.springeropen.com/articles/10.1186/2193-1801-3-723
that the surfaces of hard rocky planets in our solar system would be like the moon’s if they had no atmosphere. Given what we now know about the extent to which the moon’s surface has been shaped by collisions, I don’t know why he would question that those same impacts would have occurred on other planets as well.
===========> …AND THEN THERE’S PHYSICS
The …and Then There’s Physics column also raises several issues.
》DENSITY
The first key point made is that: “the pressure alone cannot tell you what the temperature should be; it depends also on the density”.
This point is readily addressed by noting that this paper by Robert Holmes:
Thermal Enhancement on Planetary Bodies and the Relevance of the Molar Mass Version of the Ideal Gas Law to the Null Hypothesis of Climate Change
https://www.researchgate.net/publication/324599511_Thermal_Enhancement_on_Planetary_Bodies_and_the_Relevance_of_the_Molar_Mass_Version_of_the_Ideal_Gas_Law_to_the_Null_Hypothesis_of_Climate_Change
provides a *density-based* formulation very similar to the N&Z formulation. If memory serves, I seem to recall Nikolov writing a post that addressed the mathematical equivalence of the two formulations. But even if my memory is incorrect, both formulations produce nearly identical results so they are at least functionally equivalent.
》RADIATIVE ENERGY
The remainder of the column elaborates on the reasoning from the Arthur Smith paper, which I addressed above, and makes basically the same incorrect assumption, stating: “The only way to explain why the surface radiates more energy than it gets from the Sun is because of the atmospheric greenhouse effect”.
Again the word “only”, but in a slightly different sense. As N&Z state: “the so-called ‘greenhouse back radiation’ is globally a result of the atmospheric thermal effect rather than a cause for it”.
The N&Z ATE provides a viable alternative explanation for why the surface radiates more energy than it gets from the Sun. The assumption that “only” the greenhouse effect can explain this phenomenon is just that — an assumption yet to be proven.
So, right now, there are at least two logically-viable alternative explanations for the surface radiative phenomenon. One of those two (N&Z) demonstrates very good predictive ability over a wide range of conditions.
===========> CONCLUSION
I believe I have adequately addressed every key point objecting to the Nikolov and Zeller theory from among the cited articles. That doesn’t prove the N&Z theory is correct, but it does say that it cannot be ruled out for any of the reasons given. Remaining in its favor is its strong, demonstrated quantitative predictive capability.
I do not believe that greenhouse effect theory can as yet match these quantitative results because, as far as I know, the detailed micro-physics of absorption and re-radiation as a function of atmospheric density, for different GHGs, has not yet been worked out to a sufficient degree of specificity.
_______________
RC,
N&Z’s theory is the complete opposite of strongly predictive. It uses multiple arbitrary parameters to fit a curve with four data points. It doesn’t predict anything. That right there is sufficient, IMO, to disregard it. The fact that you still strongly defend it is sufficient for me to ignore further posts by you. You are either completely clueless or a tr011. Goodby.
==
Just for the record:
1. Certainly not a troll.
2. Quite probably not completely clueless, either. 😉
For the sake of accuracy:
— six (not four) data points were fitted
— 4 arbitrary parameters were tuned for the fitting
plus, there was an important fitting constraint that makes things much more difficult:
— monotonic fitting functions only!
And remember the flip side: however easy or hard it may seem to have achieved such a low overall RMS fit, the fit does not involve any GHG-related parameters, which vary substantially among earth, Venus, Mars, and Titan. If we now add a new requirement that GHG parameters *must* have a substantive overall effect within the final fit, then it appears to me that the degree of fit can only get worse, not better, within the above constraints.
Lastly, the reason I can still defend the need to give this theory some legitimate scientific consideration, as opposed to just dismissing it out of hand with a few good laughs, is that I have studied quite a few different critiques of the theory that have attempted to put it out of its misery, so to speak. Oddly, not a single one that I have seen has pointed out an actual physics flaw with the N&Z theory that could stand up under scrutiny.
I say oddly, because if the theory is so nonsensical as you assert, it ought to be extremely easy for physicists to point out a demonstrable physics error that cannot be easily refuted by the theory’s proponents. Why do you think this task is proving to be so difficult to accomplish?
For someone with zero knowledge of the physics of atmospheric radiation, and zero knowledge of the last 100 years of the history of what atmospheric physicists figured out.. N&Z will appear very convincing.
After all, they don’t know anything about the subject. Large audience with equally no knowledge.
“None of us know’d nothing, so we all sat round and teached each other.”
I think that’s from an old joke about the Quakers but it’ll do for N&Z.
RationalClimate,
I’m sure you’re correct. As far as you know.
If we take out that caveat you are completely wrong.
“After all, as far as I know the motions of the planets from this theory of gravity has not yet been worked out to a sufficient degree..” Oh, wait.. [parody over]
You can see the maths of atmospheric radiation at Understanding Atmospheric Radiation and the “Greenhouse” Effect – Part Six – The Equations.
And you can see an example of theory vs experiment at Theory and Experiment – Atmospheric Radiation
Thank you. Very much. I want to learn more and will be studying both links.
And yes, I do try to be very careful with the wording of my statements.
I want to compliment you on your Part 6 column.
You hit the nail on the head when you wrote:
“Many of these blog articles review simple explanations of how it is possible for atmospheric radiative effects to increase the surface temperature … As a result many people are confused and imagine that climate science hasn’t got past “first base” with how radiation interacts with atmospheric gases.”
Those other blog articles do a considerable disservice when they don’t alert the readers that the explanation they present is *really* simple, and also don’t alert readers where to look for a more complete one.
But that isn’t the worst aspect of the damage done to the credibility of legitimate climate science by those various simplistic blog presentations: that comes from so many *conflicting* simplistic representations of the greenhouse effect. Is it any wonder that the person on the street looks askance when multiple blogs purporting to represent established climate science each provide differing explanations for the single most basic aspect of AGW?
This is why your site is like a breath of fresh air wrt the ongoing debate. Your stated purpose here:
“This article will try to “bridge the gap” between the over-simplified models and the very detailed theory.”
represents something desperately needed to help elevate the level of debate between the public and climate scientists. You have done a masterful job at achieving your purpose.
Thanks for the great work you are doing.
_____
Just as an aside, FWIW: If you were inclined to tweak the column to improve its clarity for readers, I would add some words between eq. 10b and eq. 11.
I could neither catch the motivation for that step nor the mechanics of how the substitution was accomplished.
I stand corrected in that my “micro-physics, etc.” comment was incorrect as written. However, this sentence:
“If you read back through the explanation, as it becomes clearer you will see that you need to know the quantity of CO2, water vapor and other trace gases at each height. And that you need to know the temperature at each height in the atmosphere.”
from your column:
Theory and Experiment – Atmospheric Radiation
reminded me of the thoughts that had been in the back of my mind when I wrote that.
The point I had been mulling over was that using GHE theory to construct an accurate estimator for earth’s near surface temperature — which I assumed could be done in principle — would in practice require quite a bit of input information, just as your sentence indicates, in order to properly drive that calculation.
Perhaps I was wrong in that expectation. Perhaps a “good enough” temperature estimator based on GHE can be constructed from more macro planetary parameters such as effective radiating layer or effective radiating temperature, or the like. But, as I understand it, even those types of explanations still require knowing the lapse rate a-priori.
Also, I haven’t happened to have seen any of those ERL explanations that explicitly invoke the radiative physics equations, such as you use here, to show why an effective radiating layer altitude based on where radiation predominantly escapes to space must *necessarily* match the altitude where the atmospheric temperature is exactly what it needs to be in order to produce the +33°C of additional warming that is attributed to earth’s GHE. For that matter, I haven’t come across any definitive assertion for whether or not these two differently-defined ERLs do, in fact, have the same altitude for earth. Even if they match on earth, do they also match on Venus?
The contrast I was trying to highlight was that N&Z empirical estimator formula *did* manage to use *only* basic macro physics parameters in order to produce reasonably accurate estimates.
I can liken their empirical-only work to the diffusivity approximation that you described. There may be no theoretical physics in it, but it certainly is useful for its purpose. Apart from that, it also motivated Nikolov and Zeller to work on a corresponding theory.
You decided to link me (on Twitter) to your comments here, RationalClimate, as if this is an example of you making cogent points on climate science:
So I just want people to be aware that you named yourself “notGHGs” on Twitter, claiming that anthropogenic greenhouse gases were not driving climate. Yet it turned out you didn’t know what greenhouse gases (GHGs) were; you thought that it was gases like nitrogen and argon that were visible to IR photons:
https://twitter.com/robhon_/status/1208162592939577344
So you distorted the basics.